In situ determination of NaCl-H2O isochores up to 900 oC and 1.2 GPa in a hydrothermal diamond-anvil cell
Affiliations | Corresponding Author | Cite as | Funding information- Share this article




Article views:507Cumulative count of HTML views and PDF downloads.
- Download Citation
- Rights & Permissions
top
Abstract
Li, S.H., Chou, I-M. (2022) Refinement of the α-β quartz phase boundary based on in situ Raman spectroscopy measurements in hydrothermal diamond-anvil cell and an evaluated equation of state of pure H2O. Journal of Raman Spectroscopy 53, 1471–1482. https://doi.org/10.1002/jrs.6367
). The refined isochores fitted with our data are expressed by: P (bar) = A1 + A2 × T (oC) and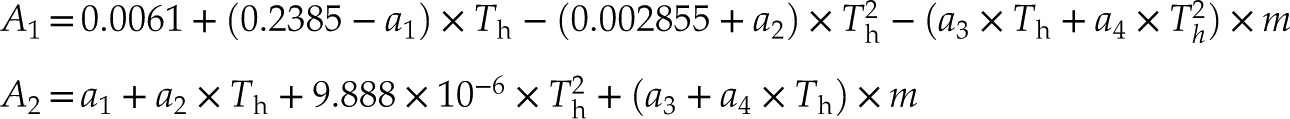
where m is the NaCl molality (mole/kg H2O), Th (°C) is the liquid-vapour homogenisation (to the liquid phase) temperature, and a1, a2, a3, and a4 are constants (27.21, −0.05956, −0.3095, and 0.003232, respectively). The isochores have better applicability for the salinity range of 5−25 wt. % NaCl, 100 oC < Th <450 oC, and P−T range up to ∼1.2 GPa and ∼900 oC. Compared with previous data, these isochores are more precise above 600 MPa, and are particularly suitable for the geological applications involving saline fluids in the deep Earth.
Figures
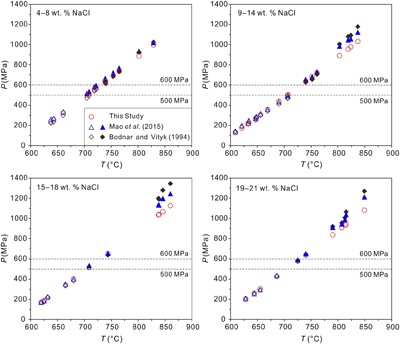 Figure 1 Comparisons of pressures at the measured α-β quartz phase transition temperatures (Ttrs). Plotted are the α-β quartz phase transition pressures (Ptrs), which were calculated from the equation of Li and Chou (2022). Other corresponding pressures were calculated from the isochores reported by Bodnar and Vityk (1994) and Mao et al. (2015) and shown by the open symbols below the 600 and 500 MPa isobars, respectively; the extrapolated pressures above the two isobars are shown by the solid symbols. All data are listed in Table S-1. | 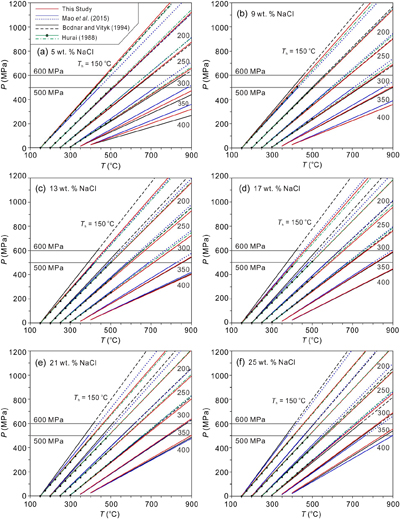 Figure 2 Comparisons of NaCl-H2O isochores derived from our experimental data (red lines), those from Bodnar and Vityk (1994; black solid lines with dashed extrapolations above 600 MPa) and Mao et al. (2015; blue lines with dotted extrapolations above 500 MPa), and isochores (green lines with dash-dotted extrapolations above 500 MPa and 500 °C) linearly fitted with data from Hurai (1988; black circles). The homogenisation temperatures (Ths) are marked. | 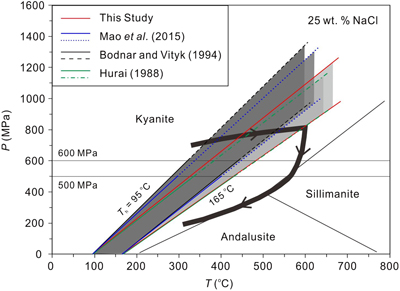 Figure 3 Application of NaCl-H2O isochores obtained in this study for the determination of the peak metamorphic conditions in Prince Rupert of the Coast Mountain, British Columbia, Canada. Hurai (1989) reported that the fluid inclusions (FIs) in quartz in this metamorphic belt have salinities of ∼25 wt. % NaCl with Ths (to L) of 95−165 oC. The P−T fields (four shaded areas) defined by the FI isochores from Hurai (1988), Mao et al. (2015) and Bodnar and Vityk (1994), and this study are compared with the metamorphic P−T path (thick line with arrows) derived from associated mineral assemblages (Crawford et al., 1987). The lines and symbols are the same as those in Figure 2. |
| Figure 1 | Figure 2 | Figure 3 |
top
Introduction
NaCl-H2O solutions exist widely in geologic environments. The properties of the pressure, volume, temperature, and composition (PVTX) of the binary system have been widely investigated to interpret the rock- and ore-forming conditions, and quantify mass transfer in many geological settings, such as subduction zones (Mantegazzi et al., 2013
Mantegazzi, D., Sanchez-Valle, C., Driesner, T. (2013) Thermodynamic properties of aqueous NaCl solutions to 1073 K and 4.5 GPa, and implications for dehydration reactions in subducting slabs. Geochimica et Cosmochimica Acta 121, 263–290. https://doi.org/10.1016/j.gca.2013.07.015
). Empirical and theoretical models have been published to describe the PVTX properties of NaCl-H2O (e.g., Bodnar and Vityk, 1994Bodnar, R.J., Vityk, M.O. (1994) Interpretation of Microthermometric data for H2O-NaCl fluid inclusions. In: Vivo, B.D., Frezzoti, M.L. (Eds.) Fluid Inclusions in Minerals: Methods and Applications. Virginia Tech., Blacksburg, VA. 117–130.
). However, most PVTX models for NaCl-H2O are applicable under low temperature (e.g., ≤700 oC) and pressure (e.g., ≤600 MPa) conditions (e.g., Bodnar and Sterner, 1987Bodnar, R.J., Sterner, S.M. (1987) Synthetic fluid inclusions. In: Ulmer, G.C., Barnes, H.L. (Eds.) Hydrothermal Experimental Techniques, Wiley-Interscience, New York. 423–457.
; Mao et al., 2015Mao, S., Hu, J., Zhang, Y., Meng, X.L. (2015) A predictive model for the PVTX properties of CO2–H2O–NaCl fluid mixture up to high temperature and high pressure. Applied Geochemistry 54, 54–64. https://doi.org/10.1016/j.apgeochem.2015.01.003
). This is mainly because these PVTX properties were derived from experimental results, such as those obtained with synthetic fluid inclusion (SFI) methods, using pressure vessels operated under relatively low P−T conditions (Gehrig, 1980Gerhig, M. (1980) Phasengleichgewichte und PVT-Daten temiirer Mischungen aus Wasser, Kohlendioxid und Natriumchlorid bis 3 kbar und 550°C. Ph.D. dissertation, Universitat Karlsruhe, 109p.
; Zhang and Frantz, 1987Zhang, Y.G., Frantz, J.D. (1987) Determination of the homogenization temperatures and densities of supercritical fluids in the system NaCl-KCl-CaCl2-H2O using synthetic fluid inclusions. Chemical Geology 64, 335–350. https://doi.org/10.1016/0009-2541(87)90012-X
; Bodnar and Vityk, 1994Bodnar, R.J., Vityk, M.O. (1994) Interpretation of Microthermometric data for H2O-NaCl fluid inclusions. In: Vivo, B.D., Frezzoti, M.L. (Eds.) Fluid Inclusions in Minerals: Methods and Applications. Virginia Tech., Blacksburg, VA. 117–130.
). Moreover, many PVTX models were built by using the equation of state (EoS) of H2O suggested by Haar et al. (1984)Haar, L., Gallagher, J.S., Kell, G.S. (1984) NBS/NRC steam tables: thermodynamic and transport properties and computer programs for vapor and liquid states of water in SI units. Hemisphere Publishing Corp, Washington, D.C.
(e.g., Driesner, 2007Driesner, T. (2007) The system H2O–NaCl. Part II: Correlations for molar volume, enthalpy, and isobaric heat capacity from 0 to 1000 oC, 1 to 5000 bars, and 0 to 1 XNaCl. Geochimica et Cosmochimica Acta 71, 4902–4919. https://doi.org/10.1016/j.gca.2007.05.026
), which has been considered to have lower accuracy than other available models at pressures >600 MPa (e.g., Li and Chou, 2022Li, S.H., Chou, I-M. (2022) Refinement of the α-β quartz phase boundary based on in situ Raman spectroscopy measurements in hydrothermal diamond-anvil cell and an evaluated equation of state of pure H2O. Journal of Raman Spectroscopy 53, 1471–1482. https://doi.org/10.1002/jrs.6367
). Consequently, most current available EoSs of H2O-NaCl are only applicable to the upper crustal P−T conditions and thus unsuitable for describing geological processes in lower crustal conditions, such as those involving the saline aqueous fluids released from subduction slabs where the pressure may become much higher than 600 MPa (e.g., Kawamoto et al., 2018Kawamoto, T., Hertwig, A., Schertl, H.-P., Maresch, W.V. (2018) Fluid inclusions in jadeitite and jadeite-rich rock from serpentinite mélanges in northern Hispaniola: Trapped ambient fluids in a cold subduction channel. Lithos 308–309, 227–241. https://doi.org/10.1016/j.lithos.2018.02.024
).In order to experimentally model such high P−T conditions, the hydrothermal diamond-anvil cell (HDAC; Bassett et al., 1993
Bassett, W.A., Shen, A.H., Bucknum, M., Chou, I-M. (1993) A new diamond anvil cell for hydrothermal studies to 2.5 GPa and from –190 to 1200 oC. Review of scientific instruments 64, 2340–2345. https://doi.org/10.1063/1.1143931
), is a good option. It can potentially yield a sample chamber with a constant volume during an experiment at pressures up to 2.5 GPa and temperatures from −190 °C to 1200 °C (Bassett et al., 1993Bassett, W.A., Shen, A.H., Bucknum, M., Chou, I-M. (1993) A new diamond anvil cell for hydrothermal studies to 2.5 GPa and from –190 to 1200 oC. Review of scientific instruments 64, 2340–2345. https://doi.org/10.1063/1.1143931
), making it excellent to measure the PVTX properties or isochores of fluids under wide P−T conditions. By using the HDAC to measure the PVTX properties of a fluid, it is crucial to determine the pressure value at a set temperature in a homogenous fluid phase inside the sample chamber (Figure S-1a). Previously, pressure sensors based on shifts of Raman or fluorescence lines in some minerals or materials (e.g., quartz and ruby) were commonly used in HDAC experiments, despite their large associated uncertainties (Schmidt and Ziemann, 2000Schmidt, C., Ziemann, M.A. (2000) In-situ Raman spectroscopy of quartz: A pressure sensor for hydrothermal diamond-anvil cell experiments at elevated temperatures. American Mineralogist 85, 1725–1734. https://doi.org/10.2138/am-2000-11-1216
). By using these pressure sensors, Mantegazzi et al. (2013)Mantegazzi, D., Sanchez-Valle, C., Driesner, T. (2013) Thermodynamic properties of aqueous NaCl solutions to 1073 K and 4.5 GPa, and implications for dehydration reactions in subducting slabs. Geochimica et Cosmochimica Acta 121, 263–290. https://doi.org/10.1016/j.gca.2013.07.015
used a diamond-anvil cell to determine the PVTX properties of NaCl-H2O solutions at 0.5–4.5 GPa and ≤400 °C (extrapolated up to 800 oC).To obtain NaCl-H2O isochore data with high precision in a wide PVTX range through HDAC experiments, this study uses the α-β quartz phase transition P−T boundary as the pressure calibrant (Figure S-1a), as done by Shen et al. (1993)
Shen, A.H., Bassett, W.A., Chou, I-M. (1993) The alpha-beta quartz transition at high temperatures and pressures in a diamond-anvil cell by laser interferometry. American Mineralogist 78, 694–698.
. In HDAC experiments, the α-β quartz phase transition temperature (Ttr) can be measured by optical observation of interference fringes (Shen et al., 1993Shen, A.H., Bassett, W.A., Chou, I-M. (1993) The alpha-beta quartz transition at high temperatures and pressures in a diamond-anvil cell by laser interferometry. American Mineralogist 78, 694–698.
) or the Raman shifts of the quartz 464 cm−1 band (Schmidt and Ziemann, 2000Schmidt, C., Ziemann, M.A. (2000) In-situ Raman spectroscopy of quartz: A pressure sensor for hydrothermal diamond-anvil cell experiments at elevated temperatures. American Mineralogist 85, 1725–1734. https://doi.org/10.2138/am-2000-11-1216
), with rigourous experimental conditions but large uncertainties (Li and Chou, 2022Li, S.H., Chou, I-M. (2022) Refinement of the α-β quartz phase boundary based on in situ Raman spectroscopy measurements in hydrothermal diamond-anvil cell and an evaluated equation of state of pure H2O. Journal of Raman Spectroscopy 53, 1471–1482. https://doi.org/10.1002/jrs.6367
). Recently, Li and Chou (2022)Li, S.H., Chou, I-M. (2022) Refinement of the α-β quartz phase boundary based on in situ Raman spectroscopy measurements in hydrothermal diamond-anvil cell and an evaluated equation of state of pure H2O. Journal of Raman Spectroscopy 53, 1471–1482. https://doi.org/10.1002/jrs.6367
found that the abrupt change in the Raman shift of the quartz 128 cm−1 band is much more sensitive and precise than that of the 464 cm−1 band during heating for the detection and measurement of the Ttr, particularly under high P conditions (Figure S-1b); a new α-β quartz P−T boundary with high precision was redefined by Li and Chou (2022)Li, S.H., Chou, I-M. (2022) Refinement of the α-β quartz phase boundary based on in situ Raman spectroscopy measurements in hydrothermal diamond-anvil cell and an evaluated equation of state of pure H2O. Journal of Raman Spectroscopy 53, 1471–1482. https://doi.org/10.1002/jrs.6367
. Moreover, a cooling system for HDAC was designed (Li et al., 2020Li, J.K., Chou, I. M., Bassett, W.A., Wang, X. (2020) A new type of hydrothermal diamond-anvil cell with cooling system. Review of Scientific Instruments 91, 053104. https://doi.org/10.1063/1.5143596
), which can be used to determine the true salinities of the loaded H2O-NaCl solutions through ice melting temperatures (Tices). This prevents an erroneous assumption that the salinity of the prepared H2O-NaCl solution is the true salinity of the loaded fluid, ignoring the effect of unavoidable evaporation of water and the corresponding increase in the salinity during loading (Li et al., 2020Li, J.K., Chou, I. M., Bassett, W.A., Wang, X. (2020) A new type of hydrothermal diamond-anvil cell with cooling system. Review of Scientific Instruments 91, 053104. https://doi.org/10.1063/1.5143596
). These new experimental procedures to measure the pressure and salinity can be applied to obtain fluid isochores with high precisions, especially at elevated temperatures and pressures. Therefore, here we loaded NaCl-H2O solutions in an HDAC sample chamber together with a chip of natural quartz (Figure S-2) to measure NaCl-H2O isochores with a Raman spectrometer.top
Experimental Results
Experimental details are provided in the Supplementary Information (Experimental Methods). A total of 53 experiments with P−T range up to 1.2 GPa and 900 °C have been conducted with HDAC in this study. In each experiment, the Tice, Ttr, and liquid−vapour homogenisation (to liquid phase) temperature (Th) of the sample fluid were recorded (Table S-1). During heating, the Raman shifts of the 128 cm−1 Raman band of α-quartz in the sample chamber were collected in each experiment to determine Ttrs (Figure S-1b). Subsequently, the α-β quartz phase transition pressure (Ptr) was calculated from Ttr according to the refined α-β quartz P−T boundary of Li and Chou (2022)
Li, S.H., Chou, I-M. (2022) Refinement of the α-β quartz phase boundary based on in situ Raman spectroscopy measurements in hydrothermal diamond-anvil cell and an evaluated equation of state of pure H2O. Journal of Raman Spectroscopy 53, 1471–1482. https://doi.org/10.1002/jrs.6367
.Each isochore of NaCl-H2O in our experiment is established through two P−T points; one is the Ph−Th of the NaCl-H2O system in the HDAC sample chamber, and the other is Ptr−Ttr described above (Figure S-1a). The Ph is the liquid-vapour homogenisation (to the liquid phase) pressure for the sample NaCl-H2O fluid calculated from the measured Th by using the equation of Bodnar (1983)
Bodnar, R.J. (1983) A method of calculating fluid inclusion volumes based on vapor bubble diameters and P-V-T-X properties of inclusion fluids. Economic Geology 78, 535–542. https://doi.org/10.2113/gsecongeo.78.3.535
. All the P−T data of the NaCl-H2O isochores are presented in Table S-1 and Figure 1.
Figure 1 Comparisons of pressures at the measured α-β quartz phase transition temperatures (Ttrs). Plotted are the α-β quartz phase transition pressures (Ptrs), which were calculated from the equation of Li and Chou (2022)
Li, S.H., Chou, I-M. (2022) Refinement of the α-β quartz phase boundary based on in situ Raman spectroscopy measurements in hydrothermal diamond-anvil cell and an evaluated equation of state of pure H2O. Journal of Raman Spectroscopy 53, 1471–1482. https://doi.org/10.1002/jrs.6367
. Other corresponding pressures were calculated from the isochores reported by Bodnar and Vityk (1994)Bodnar, R.J., Vityk, M.O. (1994) Interpretation of Microthermometric data for H2O-NaCl fluid inclusions. In: Vivo, B.D., Frezzoti, M.L. (Eds.) Fluid Inclusions in Minerals: Methods and Applications. Virginia Tech., Blacksburg, VA. 117–130.
and Mao et al. (2015)Mao, S., Hu, J., Zhang, Y., Meng, X.L. (2015) A predictive model for the PVTX properties of CO2–H2O–NaCl fluid mixture up to high temperature and high pressure. Applied Geochemistry 54, 54–64. https://doi.org/10.1016/j.apgeochem.2015.01.003
and shown by the open symbols below the 600 and 500 MPa isobars, respectively; the extrapolated pressures above the two isobars are shown by the solid symbols. All data are listed in Table S-1 .top
Discussion
Isochores fitted with the experimental data. To facilitate the interpolation of our experimental results, we used the equation formats provided by Zhang and Frantz (1987)
Zhang, Y.G., Frantz, J.D. (1987) Determination of the homogenization temperatures and densities of supercritical fluids in the system NaCl-KCl-CaCl2-H2O using synthetic fluid inclusions. Chemical Geology 64, 335–350. https://doi.org/10.1016/0009-2541(87)90012-X
to fit the H2O-NaCl isochores determined with (Th, Ph) and (Ttr, Ptr) listed in Table S-1. This is because their equations were built with the liquid-vapour homogenisation T and P and the corresponding entrapment P−T conditions of SFIs, which are similar to the data groups (Th, Ph and Ttr, Ptr) collected in this study (Table S-1). Moreover, their equations can accurately describe PVTX data from many experiments, as commented by Brown (1989)Brown, P.E. (1989) Flincor: A microcomputer program for the reduction and investigation of fluid-inclusion data. American Mineralogist 74, 1390–1393.
. Accordingly, the H2O-NaCl isochores of this study can be fitted by the following equation with R2 = 0.991:Eq. 1
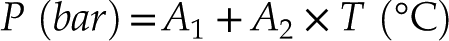
where
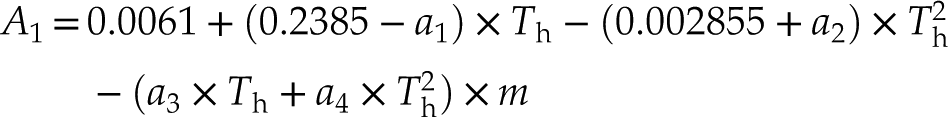
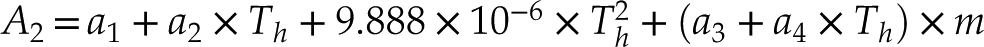
The constants A1 and A2 are functions of Th (°C) of the fluid inclusions or NaCl-H2O solution in the HDAC sample chamber and salinity (m, the NaCl molality in aqueous solution). The parameters a1, a2, a3, and a4 are 27.21, −0.05956, −0.3095, and 0.003232, respectively. The average errors between the values calculated from Equation 1 and the experimental data for Ptr and isochore slopes are 3.7 % and 3.9 %, respectively (Table S-1). These fitting errors among NaCl-H2O fluids with low to high salinities are consistent (Table S-1), and they are different from those of Zhang and Frantz (1987)
Zhang, Y.G., Frantz, J.D. (1987) Determination of the homogenization temperatures and densities of supercritical fluids in the system NaCl-KCl-CaCl2-H2O using synthetic fluid inclusions. Chemical Geology 64, 335–350. https://doi.org/10.1016/0009-2541(87)90012-X
which contain large errors for high density NaCl-H2O solutions, as pointed out by Brown (1989)Brown, P.E. (1989) Flincor: A microcomputer program for the reduction and investigation of fluid-inclusion data. American Mineralogist 74, 1390–1393.
, indicating a better fitting of Equation 1 in this study. Considering the P−T range of our experiments, Equation 1 is considered to have better applicability for solutions with salinity and Th ranges of 5−21 wt. % NaCl and 100−450 °C, respectively.Comparisons of experimental data with previous studies. The experimental data of this study are compared with those derived from Bodnar and Vityk (1994)
Bodnar, R.J., Vityk, M.O. (1994) Interpretation of Microthermometric data for H2O-NaCl fluid inclusions. In: Vivo, B.D., Frezzoti, M.L. (Eds.) Fluid Inclusions in Minerals: Methods and Applications. Virginia Tech., Blacksburg, VA. 117–130.
and Mao et al. (2015)Mao, S., Hu, J., Zhang, Y., Meng, X.L. (2015) A predictive model for the PVTX properties of CO2–H2O–NaCl fluid mixture up to high temperature and high pressure. Applied Geochemistry 54, 54–64. https://doi.org/10.1016/j.apgeochem.2015.01.003
(Figure 1). The model of Bodnar and Vityk (1994)Bodnar, R.J., Vityk, M.O. (1994) Interpretation of Microthermometric data for H2O-NaCl fluid inclusions. In: Vivo, B.D., Frezzoti, M.L. (Eds.) Fluid Inclusions in Minerals: Methods and Applications. Virginia Tech., Blacksburg, VA. 117–130.
, determined using the SFI method, is applicable at ≤600 MPa, and has been widely used to interpret the PVTX properties of geological fluids (e.g., Sullivan et al., 2022Sullivan N.A., Zoltán Z., Brenan J.M., Hinde J.C., Yin Y.W. (2022) The solubility of gold and palladium in magmatic brines: Implications for PGE enrichment in mafic-ultramafic and porphyry environments. Geochimica et Cosmochimica Acta 316, 230–252. https://doi.org/10.1016/j.gca.2021.09.010
). Mao’s model (Mao et al., 2015Mao, S., Hu, J., Zhang, Y., Meng, X.L. (2015) A predictive model for the PVTX properties of CO2–H2O–NaCl fluid mixture up to high temperature and high pressure. Applied Geochemistry 54, 54–64. https://doi.org/10.1016/j.apgeochem.2015.01.003
), as a representative thermodynamic model, works up to 1000 °C and 500 MPa. The agreements, among the Ptr obtained from our experimental data and those from Bodnar and Vityk (1994)Bodnar, R.J., Vityk, M.O. (1994) Interpretation of Microthermometric data for H2O-NaCl fluid inclusions. In: Vivo, B.D., Frezzoti, M.L. (Eds.) Fluid Inclusions in Minerals: Methods and Applications. Virginia Tech., Blacksburg, VA. 117–130.
and Mao et al. (2015)Mao, S., Hu, J., Zhang, Y., Meng, X.L. (2015) A predictive model for the PVTX properties of CO2–H2O–NaCl fluid mixture up to high temperature and high pressure. Applied Geochemistry 54, 54–64. https://doi.org/10.1016/j.apgeochem.2015.01.003
below 600 MPa for the measured Ttrs (Figure 1), demonstrate the reliability of our experimental method and results. However, the deviations of Ptr values obtained in our experiments from those extrapolated from the previous isochores, particularly Bodnar and Vityk (1994)Bodnar, R.J., Vityk, M.O. (1994) Interpretation of Microthermometric data for H2O-NaCl fluid inclusions. In: Vivo, B.D., Frezzoti, M.L. (Eds.) Fluid Inclusions in Minerals: Methods and Applications. Virginia Tech., Blacksburg, VA. 117–130.
, are evident above 600 MPa, and they increase with salinity (Figure 1).The isochores of NaCl-H2O solutions calculated with Equation 1 in this study were primarily compared with those derived from Hurai (1988)
Hurai, V. (1988) P–V–T–X tables of water and 1–25 weight percent NaCl-H2O solutions to 500 °C and 500 × 105 Pa. Acta Geologica et Geographica Universitatis Comenianae 44, 101–135.
, Bodnar and Vityk (1994)Bodnar, R.J., Vityk, M.O. (1994) Interpretation of Microthermometric data for H2O-NaCl fluid inclusions. In: Vivo, B.D., Frezzoti, M.L. (Eds.) Fluid Inclusions in Minerals: Methods and Applications. Virginia Tech., Blacksburg, VA. 117–130.
, and Mao et al. (2015)Mao, S., Hu, J., Zhang, Y., Meng, X.L. (2015) A predictive model for the PVTX properties of CO2–H2O–NaCl fluid mixture up to high temperature and high pressure. Applied Geochemistry 54, 54–64. https://doi.org/10.1016/j.apgeochem.2015.01.003
in Figure 2. Note that the isochores of NaCl-H2O fluids in Bodnar and Vityk (1994)Bodnar, R.J., Vityk, M.O. (1994) Interpretation of Microthermometric data for H2O-NaCl fluid inclusions. In: Vivo, B.D., Frezzoti, M.L. (Eds.) Fluid Inclusions in Minerals: Methods and Applications. Virginia Tech., Blacksburg, VA. 117–130.
derived from the SFI technology were approximated by connecting the P−T point at which the SFI was formed (Pf−Tf), and the P−T point defined by the observed liquid-vapour homogenisation (to liquid phase) T (Ph−Th), assuming the volumes of the studied SFI at these two P−T points were the same (isochoric) (Bodnar, 1995Bodnar, R.J. (1995) Experimental determination of the PVTX properties of aqueous solutions at elevated temperatures and pressures using synthetic fluid inclusions: H2O-NaCI as an example. Pure and Applied Chemistry 67, 873–880. https://doi.org/10.1351/pac199567060873
). However, it was clearly shown in Figure 17.3 of Bodnar and Sterner (1987)Bodnar, R.J., Sterner, S.M. (1987) Synthetic fluid inclusions. In: Ulmer, G.C., Barnes, H.L. (Eds.) Hydrothermal Experimental Techniques, Wiley-Interscience, New York. 423–457.
that, even for the pure H2O system, the volumes of the studied SFIs at these two P−T points were not expected to be the same for most of SFIs (Bodnar and Sterner, 1987Bodnar, R.J., Sterner, S.M. (1987) Synthetic fluid inclusions. In: Ulmer, G.C., Barnes, H.L. (Eds.) Hydrothermal Experimental Techniques, Wiley-Interscience, New York. 423–457.
; their Table 17.1). To clearly demonstrate their warning, their experimental results for SFIs trapped at 100 MPa and 300, 400, 500 and 600 °C were shown in Figure S-3 by adding the isochores based on the densities of pure H2O at these two P−T points, which were derived from the well established EoS of H2O IAPWS-95 (Wagner and Pruß, 2002Wagner, W., Pruβ, A. (2002) The IAPWS formulation 1995 for the thermodynamic properties of ordinary water substance for general and scientific use. Journal of Physical and Chemical Reference Data 31, 387–535. https://doi.org/10.1063/1.1461829
). Therefore, the isochores obtained by SFI methods should strictly be called as iso-Th lines, unless the SFI volumes are corrected. Conversely, our isochores were measured in situ, during which the volume of the HDAC sample chamber was kept constant. Accordingly, the isochores of this study with high salinities and low Ths evidently deviate from those extrapolated from Bodnar and Vityk (1994)Bodnar, R.J., Vityk, M.O. (1994) Interpretation of Microthermometric data for H2O-NaCl fluid inclusions. In: Vivo, B.D., Frezzoti, M.L. (Eds.) Fluid Inclusions in Minerals: Methods and Applications. Virginia Tech., Blacksburg, VA. 117–130.
(Figure 2), consistent with the deviations of Ptr values above 600 MPa shown in Figure 1 .
Figure 2 Comparisons of NaCl-H2O isochores derived from our experimental data (red lines), those from Bodnar and Vityk (1994
Bodnar, R.J., Vityk, M.O. (1994) Interpretation of Microthermometric data for H2O-NaCl fluid inclusions. In: Vivo, B.D., Frezzoti, M.L. (Eds.) Fluid Inclusions in Minerals: Methods and Applications. Virginia Tech., Blacksburg, VA. 117–130.
; black solid lines with dashed extrapolations above 600 MPa) and Mao et al. (2015Mao, S., Hu, J., Zhang, Y., Meng, X.L. (2015) A predictive model for the PVTX properties of CO2–H2O–NaCl fluid mixture up to high temperature and high pressure. Applied Geochemistry 54, 54–64. https://doi.org/10.1016/j.apgeochem.2015.01.003
; blue lines with dotted extrapolations above 500 MPa), and isochores (green lines with dash-dotted extrapolations above 500 MPa and 500 °C) linearly fitted with data from Hurai (1988Hurai, V. (1988) P–V–T–X tables of water and 1–25 weight percent NaCl-H2O solutions to 500 °C and 500 × 105 Pa. Acta Geologica et Geographica Universitatis Comenianae 44, 101–135.
; black circles). The homogenisation temperatures (Ths) are marked.
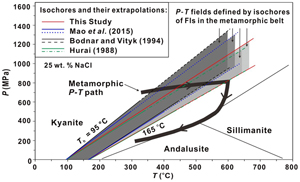
Figure 3 Application of NaCl-H2O isochores obtained in this study for the determination of the peak metamorphic conditions in Prince Rupert of the Coast Mountain, British Columbia, Canada. Hurai (1989)
Hurai, V. (1989) Basic program for interpretation of microthermometric data from H2O and H2O-NaCl fluid inclusions. Computational Geosciences 15, 135–142. https://doi.org/10.1016/0098-3004(89)90060-5
reported that the fluid inclusions (FIs) in quartz in this metamorphic belt have salinities of ∼25 wt. % NaCl with Ths (to L) of 95−165 oC. The P−T fields (four shaded areas) defined by the FI isochores from Hurai (1988)Hurai, V. (1988) P–V–T–X tables of water and 1–25 weight percent NaCl-H2O solutions to 500 °C and 500 × 105 Pa. Acta Geologica et Geographica Universitatis Comenianae 44, 101–135.
, Mao et al. (2015)Mao, S., Hu, J., Zhang, Y., Meng, X.L. (2015) A predictive model for the PVTX properties of CO2–H2O–NaCl fluid mixture up to high temperature and high pressure. Applied Geochemistry 54, 54–64. https://doi.org/10.1016/j.apgeochem.2015.01.003
and Bodnar and Vityk (1994)Bodnar, R.J., Vityk, M.O. (1994) Interpretation of Microthermometric data for H2O-NaCl fluid inclusions. In: Vivo, B.D., Frezzoti, M.L. (Eds.) Fluid Inclusions in Minerals: Methods and Applications. Virginia Tech., Blacksburg, VA. 117–130.
, and this study are compared with the metamorphic P−T path (thick line with arrows) derived from associated mineral assemblages (Crawford et al., 1987Crawford M.L., Hollister L.S., Woodsworth G.J. (1987) Crustal deformation and regional metamorphism across a terrane boundary, Coast Plutonic Complex, British Columbia. Tectonics 6, 343–361. https://doi.org/10.1029/TC006i003p00343
). The lines and symbols are the same as those in Figure 2.On the other hand, the isochores of Mao et al. (2015)
Mao, S., Hu, J., Zhang, Y., Meng, X.L. (2015) A predictive model for the PVTX properties of CO2–H2O–NaCl fluid mixture up to high temperature and high pressure. Applied Geochemistry 54, 54–64. https://doi.org/10.1016/j.apgeochem.2015.01.003
were not only fitted with the accurate EoS of H2O (IAPWS-95; Wagner and Pruß, 2002Wagner, W., Pruβ, A. (2002) The IAPWS formulation 1995 for the thermodynamic properties of ordinary water substance for general and scientific use. Journal of Physical and Chemical Reference Data 31, 387–535. https://doi.org/10.1063/1.1461829
), but also calculated with the molar volume equation of the NaCl-H2O PVTX model from Driesner (2007)Driesner, T. (2007) The system H2O–NaCl. Part II: Correlations for molar volume, enthalpy, and isobaric heat capacity from 0 to 1000 oC, 1 to 5000 bars, and 0 to 1 XNaCl. Geochimica et Cosmochimica Acta 71, 4902–4919. https://doi.org/10.1016/j.gca.2007.05.026
that was developed with several thousand data points available from previous literature, including those derived from SFIs. This could cause the isochore data of Mao et al. (2015)Mao, S., Hu, J., Zhang, Y., Meng, X.L. (2015) A predictive model for the PVTX properties of CO2–H2O–NaCl fluid mixture up to high temperature and high pressure. Applied Geochemistry 54, 54–64. https://doi.org/10.1016/j.apgeochem.2015.01.003
to be closer to ours under low Th and high salinity conditions, when compared with those of Bodnar and Vityk (1994)Bodnar, R.J., Vityk, M.O. (1994) Interpretation of Microthermometric data for H2O-NaCl fluid inclusions. In: Vivo, B.D., Frezzoti, M.L. (Eds.) Fluid Inclusions in Minerals: Methods and Applications. Virginia Tech., Blacksburg, VA. 117–130.
(Figures 1, 2c–f). Furthermore, the isochores of this study, particularly those with high salinities, agree excellently with those of Hurai (1988)Hurai, V. (1988) P–V–T–X tables of water and 1–25 weight percent NaCl-H2O solutions to 500 °C and 500 × 105 Pa. Acta Geologica et Geographica Universitatis Comenianae 44, 101–135.
, which are shown in Figure 2 by the linear regression and extrapolated lines based on the data listed in Table S-2 and shown by the black dots in Figure 2. The data in Table S-2 were derived from the listed data of Hurai (1988)Hurai, V. (1988) P–V–T–X tables of water and 1–25 weight percent NaCl-H2O solutions to 500 °C and 500 × 105 Pa. Acta Geologica et Geographica Universitatis Comenianae 44, 101–135.
, which summarized previously available data, especially those from Haas (1976)Haas, J.L. (1976) Physical properties of the coexisting phases and thermochemical properties of the H2O component in boiling NaCl solutions. United States Geology Survey Bulletin 1421-A. https://pubs.usgs.gov/bul/1421a/report.pdf
for vapour-saturated liquids, Hilbert (1979)Hilbert, R. (1979) PVT-Daten von Wasser und von wässrigen Natriumchlorid-Lösungen. PhD thesis, Universität Karlsruhe, 212p.
for densities of solutions containing up to 25 wt. % NaCl at 20–40 MPa, 200–400 °C, and Gehrig (1980)Gerhig, M. (1980) Phasengleichgewichte und PVT-Daten temiirer Mischungen aus Wasser, Kohlendioxid und Natriumchlorid bis 3 kbar und 550°C. Ph.D. dissertation, Universitat Karlsruhe, 109p.
for densities of solutions containing up to 20 wt. % NaCl at 10–300 MPa, 200–600 °C, covering 100–500 °C, ≤500 MPa and Ths of 83–325 °C. The PVT data of Hilbert (1979)Hilbert, R. (1979) PVT-Daten von Wasser und von wässrigen Natriumchlorid-Lösungen. PhD thesis, Universität Karlsruhe, 212p.
and Gehrig (1980)Gerhig, M. (1980) Phasengleichgewichte und PVT-Daten temiirer Mischungen aus Wasser, Kohlendioxid und Natriumchlorid bis 3 kbar und 550°C. Ph.D. dissertation, Universitat Karlsruhe, 109p.
were collected with volume-calibrated pressure vessels under specified P−T conditions. Representative data of Gehrig (1980)Gerhig, M. (1980) Phasengleichgewichte und PVT-Daten temiirer Mischungen aus Wasser, Kohlendioxid und Natriumchlorid bis 3 kbar und 550°C. Ph.D. dissertation, Universitat Karlsruhe, 109p.
for 20 wt. % NaCl solution are shown in Figure S-4a as an example, to show these data were excellently presented by Hurai (1988)Hurai, V. (1988) P–V–T–X tables of water and 1–25 weight percent NaCl-H2O solutions to 500 °C and 500 × 105 Pa. Acta Geologica et Geographica Universitatis Comenianae 44, 101–135.
. These features support the reliability of our isochores, implying that the isochores under our experimental conditions are approximately linear in P−T space and that the applicability of Equation 1 can be extended to the aqueous solutions containing 25 wt. % NaCl. Moreover, our isochores agree very well with those calculated from the density data reported by Pitzer et al. (1984)Pitzer, K.S., Peiper, J.C., Busey, R.H. (1984) Thermodynamic properties of aqueous sodium chloride solutions. Journal of Physical and Chemical Reference Data 13, 1–102. https://doi.org/10.1063/1.555709
and Majer et al. (1988)Majer, V., Gates, J.A., Inglese, A., Wood, R.H. (1988) Volumetric properties of aqueous NaCl solutions from 0.0025 to 5.0 mol kg1, 323 to 600 K, and 0.1 to 40 MPa. The Journal of Chemical Thermodynamics 20, 949–968. https://doi.org/10.1016/0021-9614(88)90224-8
within their rather limited applicable P−T areas (i.e. <100 or 40 MPa and <350 °C shown in Figure S-4b,c). However, deviations occur when extrapolating the isochores calculated from their data to higher P−T conditions, possibly due to the small curvature of their isochores, which are not suitable for linear extrapolations. Additionally, the PVTX models of Mantegazzi et al. (2013)Mantegazzi, D., Sanchez-Valle, C., Driesner, T. (2013) Thermodynamic properties of aqueous NaCl solutions to 1073 K and 4.5 GPa, and implications for dehydration reactions in subducting slabs. Geochimica et Cosmochimica Acta 121, 263–290. https://doi.org/10.1016/j.gca.2013.07.015
and Fowler and Sherman (2020)Fowler, S.J., Sherman, D.M. (2020) The nature of NaCl–H2O deep fluids from ab initio molecular dynamics at 0.5–4.5 GPa, 20–800 °C, and 1–14 m NaCl. Geochimica et Cosmochimica Acta 277, 243–264. https://doi.org/10.1016/j.gca.2020.03.031
are not considered for comparison here, as their isochores are only suitable for NaCl-H2O solutions with densities (primarily >1.0 kg/cm3) much higher than those in this study.Application of the isochores in the deep Earth setting. As discussed above, our isochores of the NaCl-H2O solutions are more reliable under conditions above >600 MPa. Therefore, our isochores are expected to provide better applications in lower crustal conditions. For example, the isochores of fluid inclusions in metamorphic rocks are commonly used to determine the peak metamorphic conditions in the deep Earth setting such as a subduction zone (e.g., Kawamoto et al., 2018
Kawamoto, T., Hertwig, A., Schertl, H.-P., Maresch, W.V. (2018) Fluid inclusions in jadeitite and jadeite-rich rock from serpentinite mélanges in northern Hispaniola: Trapped ambient fluids in a cold subduction channel. Lithos 308–309, 227–241. https://doi.org/10.1016/j.lithos.2018.02.024
). The western Coast Mountains of British Columbia, Canada formed during terrane accretion in the Jurassic and Cretaceous Periods (Wolf et al., 2010Wolf, D.E., Andronicos, C.L., Vervoort, J.D., Mansfield, M.R., Chardon, D. (2010) Application of Lu–Hf garnet dating to unravel the relationships between deformation, metamorphism and plutonism: An example from the Prince Rupert area, British Columbia. Tectonophysics 485, 62–77. https://doi.org/10.1016/j.tecto.2009.11.020
). The metamorphic framework in Prince Rupert of the Coast Mountains comprises schist, gneiss, and migmatite, displaying progressive regional metamorphism. In this area, the fluid inclusions in quartz contain 25 wt. % NaCl, and the observed homogenisation Ts (to liquid) were between 95 and 165 °C (Hurai, 1989Hurai, V. (1989) Basic program for interpretation of microthermometric data from H2O and H2O-NaCl fluid inclusions. Computational Geosciences 15, 135–142. https://doi.org/10.1016/0098-3004(89)90060-5
). As shown in Figure 3, the P−T field defined by the isochores of these fluid inclusions based on the models of Bodnar and Vityk (1994)Bodnar, R.J., Vityk, M.O. (1994) Interpretation of Microthermometric data for H2O-NaCl fluid inclusions. In: Vivo, B.D., Frezzoti, M.L. (Eds.) Fluid Inclusions in Minerals: Methods and Applications. Virginia Tech., Blacksburg, VA. 117–130.
and Mao et al. (2015)Mao, S., Hu, J., Zhang, Y., Meng, X.L. (2015) A predictive model for the PVTX properties of CO2–H2O–NaCl fluid mixture up to high temperature and high pressure. Applied Geochemistry 54, 54–64. https://doi.org/10.1016/j.apgeochem.2015.01.003
does not match the metamorphic P−T path derived from associated mineral assemblages (Crawford et al., 1987Crawford M.L., Hollister L.S., Woodsworth G.J. (1987) Crustal deformation and regional metamorphism across a terrane boundary, Coast Plutonic Complex, British Columbia. Tectonics 6, 343–361. https://doi.org/10.1029/TC006i003p00343
). However, the P−T field derived from our isochore model matches perfectly well with the metamorphic P−T path.The isochores of this study can also be used to infer the formation P−T conditions of melt inclusions (MIs) in plutonic rocks. A fluid subsystem inside MIs usually belongs to the NaCl-H2O system, existing as a shrinkage bubble. Its isochores are commonly used to estimate the MI entrapment pressure (Hurai et al., 2015
Hurai, V., Huraiova M., Slobodnik M., Thomas R. (2015) Geofluids—Developments in Microthermometry, Spectroscopy, Thermodynamics, and Stable Isotopes. Elsevier, Amsterdam, The Netherlands, 489p. https://doi.org/10.1016/C2014-0-03099-7
). By this method, the overestimation of the pressure values could be avoided, if our NaCl-H2O isochores rather than those extrapolated from Bodnar and Vityk (1994)Bodnar, R.J., Vityk, M.O. (1994) Interpretation of Microthermometric data for H2O-NaCl fluid inclusions. In: Vivo, B.D., Frezzoti, M.L. (Eds.) Fluid Inclusions in Minerals: Methods and Applications. Virginia Tech., Blacksburg, VA. 117–130.
and Mao et al. (2015)Mao, S., Hu, J., Zhang, Y., Meng, X.L. (2015) A predictive model for the PVTX properties of CO2–H2O–NaCl fluid mixture up to high temperature and high pressure. Applied Geochemistry 54, 54–64. https://doi.org/10.1016/j.apgeochem.2015.01.003
were used.top
Conclusions
The isochores of NaCl-H2O solutions with salinities of up to 21 wt. % NaCl (applicable up to 25 wt. % NaCl), measured in the HDAC experiments by using the re-fitted α-β quartz P−T boundary of Li and Chou (2022)
Li, S.H., Chou, I-M. (2022) Refinement of the α-β quartz phase boundary based on in situ Raman spectroscopy measurements in hydrothermal diamond-anvil cell and an evaluated equation of state of pure H2O. Journal of Raman Spectroscopy 53, 1471–1482. https://doi.org/10.1002/jrs.6367
, were extended to ∼900 oC and ∼1.2 GPa.At pressures above 600 MPa, our isochores are considered to be reliable and accurate relative to previous ones and their extrapolations, particularly those derived from analyses of SFIs. Therefore, our isochores are more suitable to be applied for the interpretations of geological processes involving NaCl-H2O fluids in the lower crust.
Our experiments also suggest a fast method for the accurate measurement of isochores of geologically important saline solutions with solutes of LiCl, NaCl, KCl, CaCl2, etc., and their mixtures by using HDAC and the newly calibrated α-β quartz P−T boundary of Li and Chou (2022)
Li, S.H., Chou, I-M. (2022) Refinement of the α-β quartz phase boundary based on in situ Raman spectroscopy measurements in hydrothermal diamond-anvil cell and an evaluated equation of state of pure H2O. Journal of Raman Spectroscopy 53, 1471–1482. https://doi.org/10.1002/jrs.6367
under wide P–T conditions.top
Ackowledgements
We would like to thank Dr. Christian Schmidt and one anonymous reviewer for constructive reviews and suggestions, Prof. Rui Sun for his help in fitting the NaCl-H2O isochores with the experimental data, and Dr. Nanfei Cheng for improving the English presentation. This study was supported by the National Natural Science Foundation of China (Grant Nos. 42330806, 41973055, and 42130109).
Editor: Anat Shahar
top
References
Bassett, W.A., Shen, A.H., Bucknum, M., Chou, I-M. (1993) A new diamond anvil cell for hydrothermal studies to 2.5 GPa and from –190 to 1200 oC. Review of scientific instruments 64, 2340–2345. https://doi.org/10.1063/1.1143931
 Show in context
Show in context In order to experimentally model such high P−T conditions, the hydrothermal diamond-anvil cell (HDAC; Bassett et al., 1993), is a good option. It can potentially yield a sample chamber with a constant volume during an experiment at pressures up to 2.5 GPa and temperatures from −190 °C to 1200 °C (Bassett et al., 1993), making it excellent to measure the PVTX properties or isochores of fluids under wide P−T conditions.
View in article
Bodnar, R.J. (1983) A method of calculating fluid inclusion volumes based on vapor bubble diameters and P-V-T-X properties of inclusion fluids. Economic Geology 78, 535–542. https://doi.org/10.2113/gsecongeo.78.3.535
 Show in context
Show in context The Ph is the liquid-vapour homogenisation (to the liquid phase) pressure for the sample NaCl-H2O fluid calculated from the measured Th by using the equation of Bodnar (1983).
View in article
Bodnar, R.J. (1995) Experimental determination of the PVTX properties of aqueous solutions at elevated temperatures and pressures using synthetic fluid inclusions: H2O-NaCI as an example. Pure and Applied Chemistry 67, 873–880. https://doi.org/10.1351/pac199567060873
 Show in context
Show in context Note that the isochores of NaCl-H2O fluids in Bodnar and Vityk (1994) derived from the SFI technology were approximated by connecting the P−T point at which the SFI was formed (Pf−Tf), and the P−T point defined by the observed liquid-vapour homogenisation (to liquid phase) T (Ph−Th), assuming the volumes of the studied SFI at these two P−T points were the same (isochoric) (Bodnar, 1995).
View in article
Bodnar, R.J., Sterner, S.M. (1987) Synthetic fluid inclusions. In: Ulmer, G.C., Barnes, H.L. (Eds.) Hydrothermal Experimental Techniques, Wiley-Interscience, New York. 423–457.
 Show in context
Show in context However, most PVTX models for NaCl-H2O are applicable under low temperature (e.g., ≤700 oC) and pressure (e.g., ≤600 MPa) conditions (e.g., Bodnar and Sterner, 1987; Mao et al., 2015).
View in article
However, it was clearly shown in Figure 17.3 of Bodnar and Sterner (1987) that, even for the pure H2O system, the volumes of the studied SFIs at these two P−T points were not expected to be the same for most of SFIs (Bodnar and Sterner, 1987; their Table 17.1).
View in article
Bodnar, R.J., Vityk, M.O. (1994) Interpretation of Microthermometric data for H2O-NaCl fluid inclusions. In: Vivo, B.D., Frezzoti, M.L. (Eds.) Fluid Inclusions in Minerals: Methods and Applications. Virginia Tech., Blacksburg, VA. 117–130.
 Show in context
Show in context Empirical and theoretical models have been published to describe the PVTX properties of NaCl-H2O (e.g., Bodnar and Vityk, 1994).
View in article
This is mainly because these PVTX properties were derived from experimental results, such as those obtained with synthetic fluid inclusion (SFI) methods, using pressure vessels operated under relatively low P−T conditions (Gehrig, 1980; Zhang and Frantz, 1987; Bodnar and Vityk, 1994).
View in article
Other corresponding pressures were calculated from the isochores reported by Bodnar and Vityk (1994) and Mao et al. (2015) and shown by the open symbols below the 600 and 500 MPa isobars, respectively; the extrapolated pressures above the two isobars are shown by the solid symbols. All data are listed in Table S-1
View in article
The experimental data of this study are compared with those derived from Bodnar and Vityk (1994) and Mao et al. (2015) (Figure 1).
View in article
The model of Bodnar and Vityk (1994), determined using the SFI method, is applicable at ≤600 MPa, and has been widely used to interpret the PVTX properties of geological fluids (e.g., Sullivan et al., 2022).
View in article
The agreements, among the Ptr obtained from our experimental data and those from Bodnar and Vityk (1994) and Mao et al. (2015) below 600 MPa for the measured Ttrs (Figure 1), demonstrate the reliability of our experimental method and results.
View in article
However, the deviations of Ptr values obtained in our experiments from those extrapolated from the previous isochores, particularly Bodnar and Vityk (1994), are evident above 600 MPa, and they increase with salinity (Figure 1).
View in article
The isochores of NaCl-H2O solutions calculated with Equation 1 in this study were primarily compared with those derived from Hurai (1988), Bodnar and Vityk (1994), and Mao et al. (2015) in Figure 2
View in article
Note that the isochores of NaCl-H2O fluids in Bodnar and Vityk (1994) derived from the SFI technology were approximated by connecting the P−T point at which the SFI was formed (Pf−Tf), and the P−T point defined by the observed liquid-vapour homogenisation (to liquid phase) T (Ph−Th), assuming the volumes of the studied SFI at these two P−T points were the same (isochoric) (Bodnar, 1995).
View in article
Accordingly, the isochores of this study with high salinities and low Ths evidently deviate from those extrapolated from Bodnar and Vityk (1994) (Figure 2), consistent with the deviations of Ptr values above 600 MPa shown in Figure 1
View in article
Comparisons of NaCl-H2O isochores derived from our experimental data (red lines), those from Bodnar and Vityk (1994; black solid lines with dashed extrapolations above 600 MPa) and Mao et al. (2015; blue lines with dotted extrapolations above 500 MPa), and isochores (green lines with dash-dotted extrapolations above 500 MPa and 500 °C) linearly fitted with data from Hurai (1988; black circles). The homogenisation temperatures (Ths) are marked.
View in article
The P−T fields (four shaded areas) defined by the FI isochores from Hurai (1988), Mao et al. (2015) and Bodnar and Vityk (1994), and this study are compared with the metamorphic P−T path (thick line with arrows) derived from associated mineral assemblages (Crawford et al., 1987).
View in article
This could cause the isochore data of Mao et al. (2015) to be closer to ours under low Th and high salinity conditions, when compared with those of Bodnar and Vityk (1994) (Figures 1, 2c–f).
View in article
As shown in Figure 3, the P−T field defined by the isochores of these fluid inclusions based on the models of Bodnar and Vityk (1994) and Mao et al. (2015) does not match the metamorphic P−T path derived from associated mineral assemblages (Crawford et al., 1987).
View in article
By this method, the overestimation of the pressure values could be avoided, if our NaCl-H2O isochores rather than those extrapolated from Bodnar and Vityk (1994) and Mao et al. (2015) were used.
View in article
Brown, P.E. (1989) Flincor: A microcomputer program for the reduction and investigation of fluid-inclusion data. American Mineralogist 74, 1390–1393.
 Show in context
Show in context Moreover, their equations can accurately describe PVTX data from many experiments, as commented by Brown (1989).
View in article
These fitting errors among NaCl-H2O fluids with low to high salinities are consistent (Table S-1), and they are different from those of Zhang and Frantz (1987) which contain large errors for high density NaCl-H2O solutions, as pointed out by Brown (1989), indicating a better fitting of Equation 1 in this study.
View in article
Crawford M.L., Hollister L.S., Woodsworth G.J. (1987) Crustal deformation and regional metamorphism across a terrane boundary, Coast Plutonic Complex, British Columbia. Tectonics 6, 343–361. https://doi.org/10.1029/TC006i003p00343
 Show in context
Show in context The P−T fields (four shaded areas) defined by the FI isochores from Hurai (1988), Mao et al. (2015) and Bodnar and Vityk (1994), and this study are compared with the metamorphic P−T path (thick line with arrows) derived from associated mineral assemblages (Crawford et al., 1987).
View in article
As shown in Figure 3, the P−T field defined by the isochores of these fluid inclusions based on the models of Bodnar and Vityk (1994) and Mao et al. (2015) does not match the metamorphic P−T path derived from associated mineral assemblages (Crawford et al., 1987).
View in article
Driesner, T. (2007) The system H2O–NaCl. Part II: Correlations for molar volume, enthalpy, and isobaric heat capacity from 0 to 1000 oC, 1 to 5000 bars, and 0 to 1 X NaCl. Geochimica et Cosmochimica Acta 71, 4902–4919. https://doi.org/10.1016/j.gca.2007.05.026
 Show in context
Show in context Moreover, many PVTX models were built by using the equation of state (EoS) of H2O suggested by Haar et al. (1984) (e.g., Driesner, 2007), which has been considered to have lower accuracy than other available models at pressures >600 MPa (e.g., Li and Chou, 2022).
View in article
On the other hand, the isochores of Mao et al. (2015) were not only fitted with the accurate EoS of H2O (IAPWS-95; Wagner and Pruß, 2002), but also calculated with the molar volume equation of the NaCl-H2O PVTX model from Driesner (2007) that was developed with several thousand data points available from previous literature, including those derived from SFIs.
View in article
Fowler, S.J., Sherman, D.M. (2020) The nature of NaCl–H2O deep fluids from ab initio molecular dynamics at 0.5–4.5 GPa, 20–800 °C, and 1–14 m NaCl. Geochimica et Cosmochimica Acta 277, 243–264. https://doi.org/10.1016/j.gca.2020.03.031
 Show in context
Show in context Additionally, the PVTX models of Mantegazzi et al. (2013) and Fowler and Sherman (2020) are not considered for comparison here, as their isochores are only suitable for NaCl-H2O solutions with densities (primarily >1.0 kg/cm3) much higher than those in this study.
View in article
Gerhig, M. (1980) Phasengleichgewichte und PVT-Daten temiirer Mischungen aus Wasser, Kohlendioxid und Natriumchlorid bis 3 kbar und 550°C. Ph.D. dissertation, Universitat Karlsruhe, 109p.
 Show in context
Show in context This is mainly because these PVTX properties were derived from experimental results, such as those obtained with synthetic fluid inclusion (SFI) methods, using pressure vessels operated under relatively low P−T conditions (Gehrig, 1980; Zhang and Frantz, 1987; Bodnar and Vityk, 1994).
View in article
The data in Table S-2 were derived from the listed data of Hurai (1988), which summarized previously available data, especially those from Haas (1976) for vapour-saturated liquids, Hilbert (1979) for densities of solutions containing up to 25 wt. % NaCl at 20–40 MPa, 200–400 °C, and Gehrig (1980) for densities of solutions containing up to 20 wt. % NaCl at 10–300 MPa, 200–600 °C, covering 100–500 °C, ≤500 MPa and Ths of 83–325 °C. The PVT data of Hilbert (1979) and Gehrig (1980) were collected with volume-calibrated pressure vessels under specified P−T conditions.
View in article
Representative data of Gehrig (1980) for 20 wt. % NaCl solution are shown in Figure S-4a as an example, to show these data were excellently presented by Hurai (1988).
View in article
Haas, J.L. (1976) Physical properties of the coexisting phases and thermochemical properties of the H2O component in boiling NaCl solutions. United States Geology Survey Bulletin 1421-A. https://pubs.usgs.gov/bul/1421a/report.pdf
 Show in context
Show in context The data in Table S-2 were derived from the listed data of Hurai (1988), which summarized previously available data, especially those from Haas (1976) for vapour-saturated liquids, Hilbert (1979) for densities of solutions containing up to 25 wt. % NaCl at 20–40 MPa, 200–400 °C, and Gehrig (1980) for densities of solutions containing up to 20 wt. % NaCl at 10–300 MPa, 200–600 °C, covering 100–500 °C, ≤500 MPa and Ths of 83–325 °C. The PVT data of Hilbert (1979) and Gehrig (1980) were collected with volume-calibrated pressure vessels under specified P−T conditions.
View in article
Haar, L., Gallagher, J.S., Kell, G.S. (1984) NBS/NRC steam tables: thermodynamic and transport properties and computer programs for vapor and liquid states of water in SI units. Hemisphere Publishing Corp, Washington, D.C.
 Show in context
Show in context Moreover, many PVTX models were built by using the equation of state (EoS) of H2O suggested by Haar et al. (1984) (e.g., Driesner, 2007), which has been considered to have lower accuracy than other available models at pressures >600 MPa (e.g., Li and Chou, 2022).
View in article
Hilbert, R. (1979) PVT-Daten von Wasser und von wässrigen Natriumchlorid-Lösungen. PhD thesis, Universität Karlsruhe, 212p.
 Show in context
Show in context The data in Table S-2 were derived from the listed data of Hurai (1988), which summarized previously available data, especially those from Haas (1976) for vapour-saturated liquids, Hilbert (1979) for densities of solutions containing up to 25 wt. % NaCl at 20–40 MPa, 200–400 °C, and Gehrig (1980) for densities of solutions containing up to 20 wt. % NaCl at 10–300 MPa, 200–600 °C, covering 100–500 °C, ≤500 MPa and Ths of 83–325 °C. The PVT data of Hilbert (1979) and Gehrig (1980) were collected with volume-calibrated pressure vessels under specified P−T conditions.
View in article
Hurai, V. (1988) P–V–T–X tables of water and 1–25 weight percent NaCl-H2O solutions to 500 °C and 500 × 105 Pa. Acta Geologica et Geographica Universitatis Comenianae 44, 101–135.
 Show in context
Show in context The isochores of NaCl-H2O solutions calculated with Equation 1 in this study were primarily compared with those derived from Hurai (1988), Bodnar and Vityk (1994), and Mao et al. (2015) in Figure 2
View in article
Comparisons of NaCl-H2O isochores derived from our experimental data (red lines), those from Bodnar and Vityk (1994; black solid lines with dashed extrapolations above 600 MPa) and Mao et al. (2015; blue lines with dotted extrapolations above 500 MPa), and isochores (green lines with dash-dotted extrapolations above 500 MPa and 500 °C) linearly fitted with data from Hurai (1988; black circles). The homogenisation temperatures (Ths) are marked.
View in article
The P−T fields (four shaded areas) defined by the FI isochores from Hurai (1988), Mao et al. (2015) and Bodnar and Vityk (1994), and this study are compared with the metamorphic P−T path (thick line with arrows) derived from associated mineral assemblages (Crawford et al., 1987).
View in article
Furthermore, the isochores of this study, particularly those with high salinities, agree excellently with those of Hurai (1988), which are shown in Figure 2 by the linear regression and extrapolated lines based on the data listed in Table S-2 and shown by the black dots in Figure 2
View in article
The data in Table S-2 were derived from the listed data of Hurai (1988), which summarized previously available data, especially those from Haas (1976) for vapour-saturated liquids, Hilbert (1979) for densities of solutions containing up to 25 wt. % NaCl at 20–40 MPa, 200–400 °C, and Gehrig (1980) for densities of solutions containing up to 20 wt. % NaCl at 10–300 MPa, 200–600 °C, covering 100–500 °C, ≤500 MPa and Ths of 83–325 °C. The PVT data of Hilbert (1979) and Gehrig (1980) were collected with volume-calibrated pressure vessels under specified P−T conditions.
View in article
Representative data of Gehrig (1980) for 20 wt. % NaCl solution are shown in Figure S-4a as an example, to show these data were excellently presented by Hurai (1988).
View in article
Hurai, V. (1989) Basic program for interpretation of microthermometric data from H2O and H2O-NaCl fluid inclusions. Computational Geosciences 15, 135–142. https://doi.org/10.1016/0098-3004(89)90060-5
 Show in context
Show in context Hurai (1989) reported that the fluid inclusions (FIs) in quartz in this metamorphic belt have salinities of ∼25 wt. % NaCl with Ths (to L) of 95−165 oC.
View in article
In this area, the fluid inclusions in quartz contain 25 wt. % NaCl, and the observed homogenisation Ts (to liquid) were between 95 and 165 °C (Hurai, 1989).
View in article
Hurai, V., Huraiova M., Slobodnik M., Thomas R. (2015) Geofluids—Developments in Microthermometry, Spectroscopy, Thermodynamics, and Stable Isotopes. Elsevier, Amsterdam, The Netherlands, 489p. https://doi.org/10.1016/C2014-0-03099-7
 Show in context
Show in context Its isochores are commonly used to estimate the MI entrapment pressure (Hurai et al., 2015).
View in article
Kawamoto, T., Hertwig, A., Schertl, H.-P., Maresch, W.V. (2018) Fluid inclusions in jadeitite and jadeite-rich rock from serpentinite mélanges in northern Hispaniola: Trapped ambient fluids in a cold subduction channel. Lithos 308–309, 227–241. https://doi.org/10.1016/j.lithos.2018.02.024
 Show in context
Show in context Consequently, most current available EoSs of H2O-NaCl are only applicable to the upper crustal P−T conditions and thus unsuitable for describing geological processes in lower crustal conditions, such as those involving the saline aqueous fluids released from subduction slabs where the pressure may become much higher than 600 MPa (e.g., Kawamoto et al., 2018).
View in article
For example, the isochores of fluid inclusions in metamorphic rocks are commonly used to determine the peak metamorphic conditions in the deep Earth setting such as a subduction zone (e.g., Kawamoto et al., 2018).
View in article
Li, J.K., Chou, I. M., Bassett, W.A., Wang, X. (2020) A new type of hydrothermal diamond-anvil cell with cooling system. Review of Scientific Instruments 91, 053104. https://doi.org/10.1063/1.5143596
 Show in context
Show in context Moreover, a cooling system for HDAC was designed (Li et al., 2020), which can be used to determine the true salinities of the loaded H2O-NaCl solutions through ice melting temperatures (Tices).
View in article
This prevents an erroneous assumption that the salinity of the prepared H2O-NaCl solution is the true salinity of the loaded fluid, ignoring the effect of unavoidable evaporation of water and the corresponding increase in the salinity during loading (Li et al., 2020).
View in article
Li, S.H., Chou, I-M. (2022) Refinement of the α-β quartz phase boundary based on in situ Raman spectroscopy measurements in hydrothermal diamond-anvil cell and an evaluated equation of state of pure H2O. Journal of Raman Spectroscopy 53, 1471–1482. https://doi.org/10.1002/jrs.6367
 Show in context
Show in context Moreover, many PVTX models were built by using the equation of state (EoS) of H2O suggested by Haar et al. (1984) (e.g., Driesner, 2007), which has been considered to have lower accuracy than other available models at pressures >600 MPa (e.g., Li and Chou, 2022).
View in article
In HDAC experiments, the α-β quartz phase transition temperature (Ttr) can be measured by optical observation of interference fringes (Shen et al., 1993) or the Raman shifts of the quartz 464 cm−1 band (Schmidt and Ziemann, 2000), with rigourous experimental conditions but large uncertainties (Li and Chou, 2022).
View in article
Recently, Li and Chou (2022) found that the abrupt change in the Raman shift of the quartz 128 cm−1 band is much more sensitive and precise than that of the 464 cm−1 band during heating for the detection and measurement of the Ttr, particularly under high P conditions (Figure S-1b); a new α-β quartz P−T boundary with high precision was redefined by Li and Chou (2022).
View in article
Subsequently, the α-β quartz phase transition pressure (Ptr) was calculated from Ttr according to the refined α-β quartz P−T boundary of Li and Chou (2022).
View in article
Plotted are the α-β quartz phase transition pressures (Ptrs), which were calculated from the equation of Li and Chou (2022).
View in article
The isochores of NaCl-H2O solutions with salinities of up to 21 wt. % NaCl (applicable up to 25 wt. % NaCl), measured in the HDAC experiments by using the re-fitted α-β quartz P−T boundary of Li and Chou (2022), were extended to ∼900 oC and ∼1.2 GPa.
View in article
Our experiments also suggest a fast method for the accurate measurement of isochores of geologically important saline solutions with solutes of LiCl, NaCl, KCl, CaCl2, etc., and their mixtures by using HDAC and the newly calibrated α-β quartz P−T boundary of Li and Chou (2022) under wide P–T conditions.
View in article
Mantegazzi, D., Sanchez-Valle, C., Driesner, T. (2013) Thermodynamic properties of aqueous NaCl solutions to 1073 K and 4.5 GPa, and implications for dehydration reactions in subducting slabs. Geochimica et Cosmochimica Acta 121, 263–290. https://doi.org/10.1016/j.gca.2013.07.015
 Show in context
Show in context The properties of the pressure, volume, temperature, and composition (PVTX) of the binary system have been widely investigated to interpret the rock- and ore-forming conditions, and quantify mass transfer in many geological settings, such as subduction zones (Mantegazzi et al., 2013).
View in article
By using these pressure sensors, Mantegazzi et al. (2013) used a diamond-anvil cell to determine the PVTX properties of NaCl-H2O solutions at 0.5–4.5 GPa and ≤400 °C (extrapolated up to 800 oC).
View in article
Additionally, the PVTX models of Mantegazzi et al. (2013) and Fowler and Sherman (2020) are not considered for comparison here, as their isochores are only suitable for NaCl-H2O solutions with densities (primarily >1.0 kg/cm3) much higher than those in this study.
View in article
Majer, V., Gates, J.A., Inglese, A., Wood, R.H. (1988) Volumetric properties of aqueous NaCl solutions from 0.0025 to 5.0 mol kg1, 323 to 600 K, and 0.1 to 40 MPa. The Journal of Chemical Thermodynamics 20, 949–968. https://doi.org/10.1016/0021-9614(88)90224-8
 Show in context
Show in context Moreover, our isochores agree very well with those calculated from the density data reported by Pitzer et al. (1984) and Majer et al. (1988) within their rather limited applicable P−T areas (i.e. <100 or 40 MPa and <350 °C shown in Figure S-4b,c).
View in article
Mao, S., Hu, J., Zhang, Y., Meng, X.L. (2015) A predictive model for the PVTX properties of CO2–H2O–NaCl fluid mixture up to high temperature and high pressure. Applied Geochemistry 54, 54–64. https://doi.org/10.1016/j.apgeochem.2015.01.003
 Show in context
Show in context However, most PVTX models for NaCl-H2O are applicable under low temperature (e.g., ≤700 oC) and pressure (e.g., ≤600 MPa) conditions (e.g., Bodnar and Sterner, 1987; Mao et al., 2015).
View in article
Other corresponding pressures were calculated from the isochores reported by Bodnar and Vityk (1994) and Mao et al. (2015) and shown by the open symbols below the 600 and 500 MPa isobars, respectively; the extrapolated pressures above the two isobars are shown by the solid symbols. All data are listed in Table S-1
View in article
The experimental data of this study are compared with those derived from Bodnar and Vityk (1994) and Mao et al. (2015) (Figure 1).
View in article
Mao’s model (Mao et al., 2015), as a representative thermodynamic model, works up to 1000 °C and 500 MPa.
View in article
The agreements, among the Ptr obtained from our experimental data and those from Bodnar and Vityk (1994) and Mao et al. (2015) below 600 MPa for the measured Ttrs (Figure 1), demonstrate the reliability of our experimental method and results.
View in article
The isochores of NaCl-H2O solutions calculated with Equation 1 in this study were primarily compared with those derived from Hurai (1988), Bodnar and Vityk (1994), and Mao et al. (2015) in Figure 2
View in article
Comparisons of NaCl-H2O isochores derived from our experimental data (red lines), those from Bodnar and Vityk (1994; black solid lines with dashed extrapolations above 600 MPa) and Mao et al. (2015; blue lines with dotted extrapolations above 500 MPa), and isochores (green lines with dash-dotted extrapolations above 500 MPa and 500 °C) linearly fitted with data from Hurai (1988; black circles). The homogenisation temperatures (Ths) are marked.
View in article
The P−T fields (four shaded areas) defined by the FI isochores from Hurai (1988), Mao et al. (2015) and Bodnar and Vityk (1994), and this study are compared with the metamorphic P−T path (thick line with arrows) derived from associated mineral assemblages (Crawford et al., 1987).
View in article
On the other hand, the isochores of Mao et al. (2015) were not only fitted with the accurate EoS of H2O (IAPWS-95; Wagner and Pruß, 2002), but also calculated with the molar volume equation of the NaCl-H2O PVTX model from Driesner (2007) that was developed with several thousand data points available from previous literature, including those derived from SFIs.
View in article
This could cause the isochore data of Mao et al. (2015) to be closer to ours under low Th and high salinity conditions, when compared with those of Bodnar and Vityk (1994) (Figures 1, 2c–f).
View in article
As shown in Figure 3, the P−T field defined by the isochores of these fluid inclusions based on the models of Bodnar and Vityk (1994) and Mao et al. (2015) does not match the metamorphic P−T path derived from associated mineral assemblages (Crawford et al., 1987).
View in article
By this method, the overestimation of the pressure values could be avoided, if our NaCl-H2O isochores rather than those extrapolated from Bodnar and Vityk (1994) and Mao et al. (2015) were used.
View in article
Pitzer, K.S., Peiper, J.C., Busey, R.H. (1984) Thermodynamic properties of aqueous sodium chloride solutions. Journal of Physical and Chemical Reference Data 13, 1–102. https://doi.org/10.1063/1.555709
 Show in context
Show in context Moreover, our isochores agree very well with those calculated from the density data reported by Pitzer et al. (1984) and Majer et al. (1988) within their rather limited applicable P−T areas (i.e. <100 or 40 MPa and <350 °C shown in Figure S-4b,c).
View in article
Schmidt, C., Ziemann, M.A. (2000) In-situ Raman spectroscopy of quartz: A pressure sensor for hydrothermal diamond-anvil cell experiments at elevated temperatures. American Mineralogist 85, 1725–1734. https://doi.org/10.2138/am-2000-11-1216
 Show in context
Show in context Previously, pressure sensors based on shifts of Raman or fluorescence lines in some minerals or materials (e.g., quartz and ruby) were commonly used in HDAC experiments, despite their large associated uncertainties (Schmidt and Ziemann, 2000).
View in article
In HDAC experiments, the α-β quartz phase transition temperature (Ttr) can be measured by optical observation of interference fringes (Shen et al., 1993) or the Raman shifts of the quartz 464 cm−1 band (Schmidt and Ziemann, 2000), with rigourous experimental conditions but large uncertainties (Li and Chou, 2022).
View in article
Shen, A.H., Bassett, W.A., Chou, I-M. (1993) The alpha-beta quartz transition at high temperatures and pressures in a diamond-anvil cell by laser interferometry. American Mineralogist 78, 694–698.
 Show in context
Show in context To obtain NaCl-H2O isochore data with high precision in a wide PVTX range through HDAC experiments, this study uses the α-β quartz phase transition P−T boundary as the pressure calibrant (Figure S-1a), as done by Shen et al. (1993).
View in article
In HDAC experiments, the α-β quartz phase transition temperature (Ttr) can be measured by optical observation of interference fringes (Shen et al., 1993) or the Raman shifts of the quartz 464 cm−1 band (Schmidt and Ziemann, 2000), with rigourous experimental conditions but large uncertainties (Li and Chou, 2022).
View in article
Sullivan N.A., Zoltán Z., Brenan J.M., Hinde J.C., Yin Y.W. (2022) The solubility of gold and palladium in magmatic brines: Implications for PGE enrichment in mafic-ultramafic and porphyry environments. Geochimica et Cosmochimica Acta 316, 230–252. https://doi.org/10.1016/j.gca.2021.09.010
 Show in context
Show in context The model of Bodnar and Vityk (1994), determined using the SFI method, is applicable at ≤600 MPa, and has been widely used to interpret the PVTX properties of geological fluids (e.g., Sullivan et al., 2022).
View in article
Wagner, W., Pruβ, A. (2002) The IAPWS formulation 1995 for the thermodynamic properties of ordinary water substance for general and scientific use. Journal of Physical and Chemical Reference Data 31, 387–535. https://doi.org/10.1063/1.1461829
 Show in context
Show in context To clearly demonstrate their warning, their experimental results for SFIs trapped at 100 MPa and 300, 400, 500 and 600 °C were shown in Figure S-3 by adding the isochores based on the densities of pure H2O at these two P−T points, which were derived from the well established EoS of H2O IAPWS-95 (Wagner and Pruß, 2002).
View in article
On the other hand, the isochores of Mao et al. (2015) were not only fitted with the accurate EoS of H2O (IAPWS-95; Wagner and Pruß, 2002), but also calculated with the molar volume equation of the NaCl-H2O PVTX model from Driesner (2007) that was developed with several thousand data points available from previous literature, including those derived from SFIs.
View in article
Wolf, D.E., Andronicos, C.L., Vervoort, J.D., Mansfield, M.R., Chardon, D. (2010) Application of Lu–Hf garnet dating to unravel the relationships between deformation, metamorphism and plutonism: An example from the Prince Rupert area, British Columbia. Tectonophysics 485, 62–77. https://doi.org/10.1016/j.tecto.2009.11.020
 Show in context
Show in context The western Coast Mountains of British Columbia, Canada formed during terrane accretion in the Jurassic and Cretaceous Periods (Wolf et al., 2010).
View in article
Zhang, Y.G., Frantz, J.D. (1987) Determination of the homogenization temperatures and densities of supercritical fluids in the system NaCl-KCl-CaCl2-H2O using synthetic fluid inclusions. Chemical Geology 64, 335–350. https://doi.org/10.1016/0009-2541(87)90012-X
 Show in context
Show in context This is mainly because these PVTX properties were derived from experimental results, such as those obtained with synthetic fluid inclusion (SFI) methods, using pressure vessels operated under relatively low P−T conditions (Gehrig, 1980; Zhang and Frantz, 1987; Bodnar and Vityk, 1994).
View in article
To facilitate the interpolation of our experimental results, we used the equation formats provided by Zhang and Frantz (1987) to fit the H2O-NaCl isochores determined with (Th, Ph) and (Ttr, Ptr) listed in Table S-1
View in article
These fitting errors among NaCl-H2O fluids with low to high salinities are consistent (Table S-1), and they are different from those of Zhang and Frantz (1987) which contain large errors for high density NaCl-H2O solutions, as pointed out by Brown (1989), indicating a better fitting of Equation 1 in this study.
View in article
top
Supplementary Information
The Supplementary Information includes:
- Experimental methods
- Tables S-1 and S-2
- Figures S-1 to S-4
- Supplementary Information References
Download the Supplementary Information (PDF)
Figures
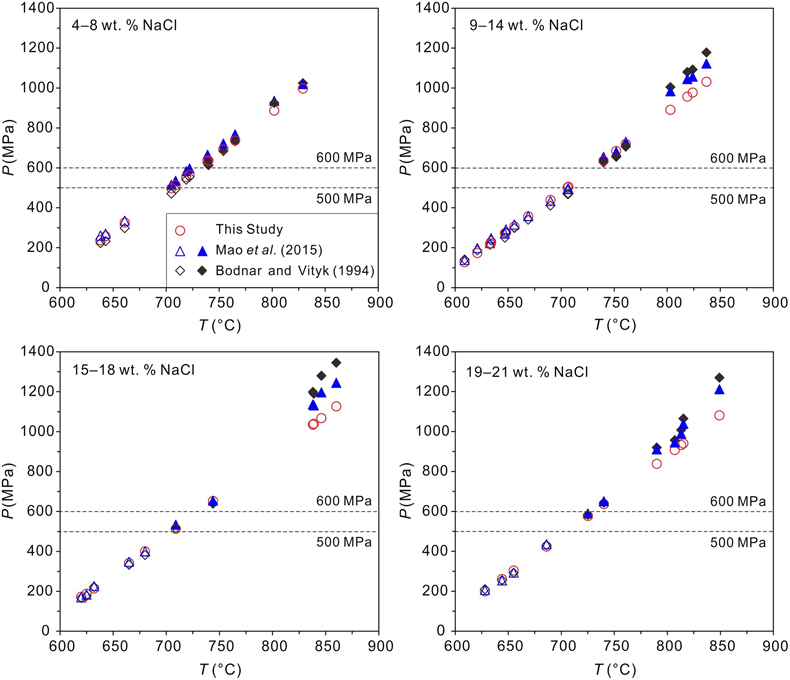
Figure 1 Comparisons of pressures at the measured α-β quartz phase transition temperatures (Ttrs). Plotted are the α-β quartz phase transition pressures (Ptrs), which were calculated from the equation of Li and Chou (2022)
Li, S.H., Chou, I-M. (2022) Refinement of the α-β quartz phase boundary based on in situ Raman spectroscopy measurements in hydrothermal diamond-anvil cell and an evaluated equation of state of pure H2O. Journal of Raman Spectroscopy 53, 1471–1482. https://doi.org/10.1002/jrs.6367
. Other corresponding pressures were calculated from the isochores reported by Bodnar and Vityk (1994)Bodnar, R.J., Vityk, M.O. (1994) Interpretation of Microthermometric data for H2O-NaCl fluid inclusions. In: Vivo, B.D., Frezzoti, M.L. (Eds.) Fluid Inclusions in Minerals: Methods and Applications. Virginia Tech., Blacksburg, VA. 117–130.
and Mao et al. (2015)Mao, S., Hu, J., Zhang, Y., Meng, X.L. (2015) A predictive model for the PVTX properties of CO2–H2O–NaCl fluid mixture up to high temperature and high pressure. Applied Geochemistry 54, 54–64. https://doi.org/10.1016/j.apgeochem.2015.01.003
and shown by the open symbols below the 600 and 500 MPa isobars, respectively; the extrapolated pressures above the two isobars are shown by the solid symbols. All data are listed in Table S-1 .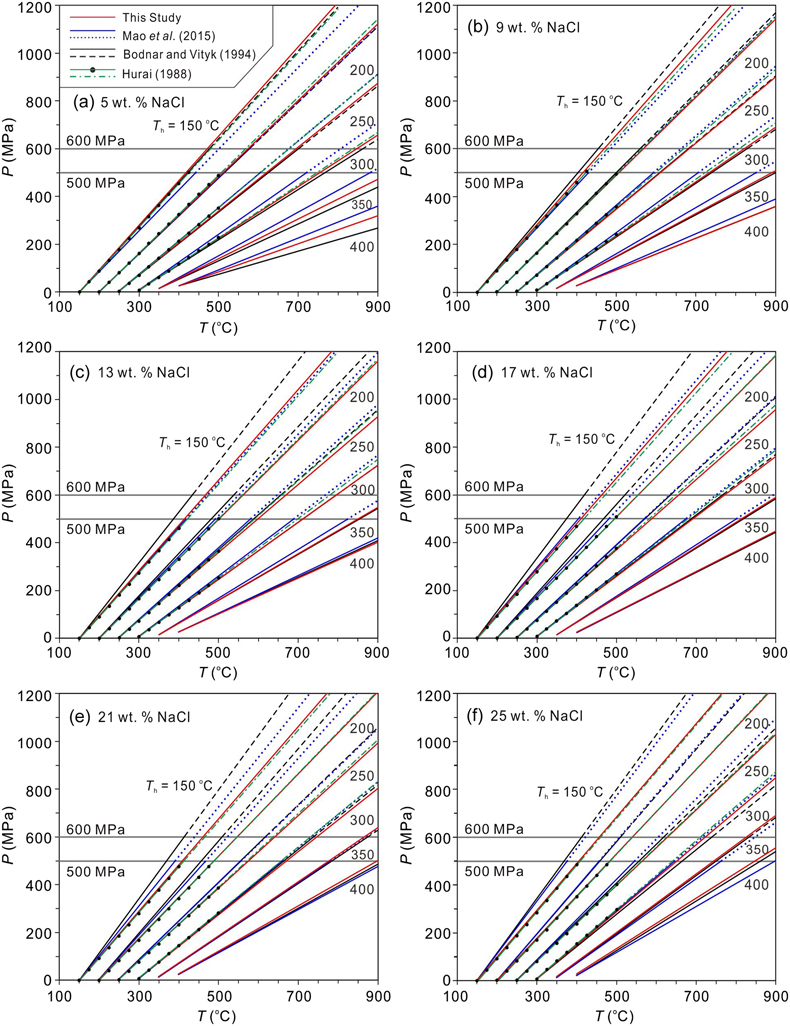
Figure 2 Comparisons of NaCl-H2O isochores derived from our experimental data (red lines), those from Bodnar and Vityk (1994
Bodnar, R.J., Vityk, M.O. (1994) Interpretation of Microthermometric data for H2O-NaCl fluid inclusions. In: Vivo, B.D., Frezzoti, M.L. (Eds.) Fluid Inclusions in Minerals: Methods and Applications. Virginia Tech., Blacksburg, VA. 117–130.
; black solid lines with dashed extrapolations above 600 MPa) and Mao et al. (2015Mao, S., Hu, J., Zhang, Y., Meng, X.L. (2015) A predictive model for the PVTX properties of CO2–H2O–NaCl fluid mixture up to high temperature and high pressure. Applied Geochemistry 54, 54–64. https://doi.org/10.1016/j.apgeochem.2015.01.003
; blue lines with dotted extrapolations above 500 MPa), and isochores (green lines with dash-dotted extrapolations above 500 MPa and 500 °C) linearly fitted with data from Hurai (1988Hurai, V. (1988) P–V–T–X tables of water and 1–25 weight percent NaCl-H2O solutions to 500 °C and 500 × 105 Pa. Acta Geologica et Geographica Universitatis Comenianae 44, 101–135.
; black circles). The homogenisation temperatures (Ths) are marked.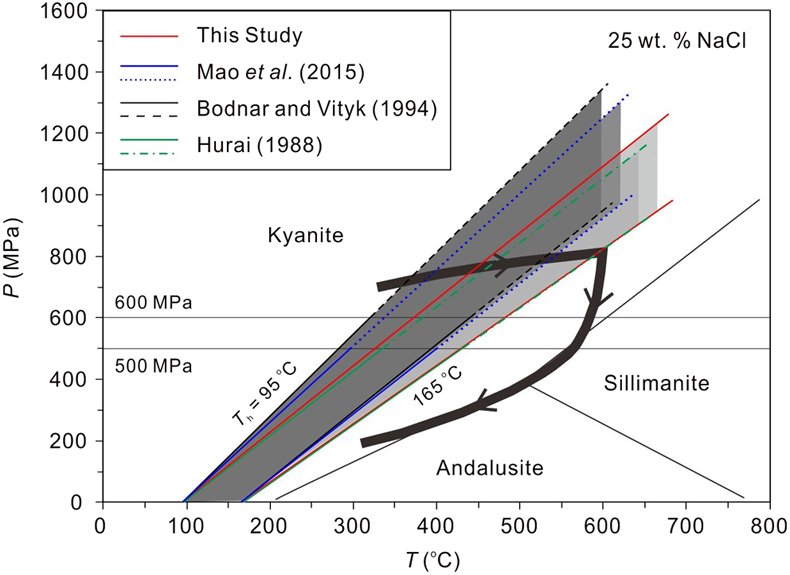
Figure 3 Application of NaCl-H2O isochores obtained in this study for the determination of the peak metamorphic conditions in Prince Rupert of the Coast Mountain, British Columbia, Canada. Hurai (1989)
Hurai, V. (1989) Basic program for interpretation of microthermometric data from H2O and H2O-NaCl fluid inclusions. Computational Geosciences 15, 135–142. https://doi.org/10.1016/0098-3004(89)90060-5
reported that the fluid inclusions (FIs) in quartz in this metamorphic belt have salinities of ∼25 wt. % NaCl with Ths (to L) of 95−165 oC. The P−T fields (four shaded areas) defined by the FI isochores from Hurai (1988)Hurai, V. (1988) P–V–T–X tables of water and 1–25 weight percent NaCl-H2O solutions to 500 °C and 500 × 105 Pa. Acta Geologica et Geographica Universitatis Comenianae 44, 101–135.
, Mao et al. (2015)Mao, S., Hu, J., Zhang, Y., Meng, X.L. (2015) A predictive model for the PVTX properties of CO2–H2O–NaCl fluid mixture up to high temperature and high pressure. Applied Geochemistry 54, 54–64. https://doi.org/10.1016/j.apgeochem.2015.01.003
and Bodnar and Vityk (1994)Bodnar, R.J., Vityk, M.O. (1994) Interpretation of Microthermometric data for H2O-NaCl fluid inclusions. In: Vivo, B.D., Frezzoti, M.L. (Eds.) Fluid Inclusions in Minerals: Methods and Applications. Virginia Tech., Blacksburg, VA. 117–130.
, and this study are compared with the metamorphic P−T path (thick line with arrows) derived from associated mineral assemblages (Crawford et al., 1987Crawford M.L., Hollister L.S., Woodsworth G.J. (1987) Crustal deformation and regional metamorphism across a terrane boundary, Coast Plutonic Complex, British Columbia. Tectonics 6, 343–361. https://doi.org/10.1029/TC006i003p00343
). The lines and symbols are the same as those in Figure 2.