Mass-dependent triple oxygen isotope variations in terrestrial materials
Affiliations | Corresponding Author | Cite as | Funding information- Share this article





Article views:5,646Cumulative count of HTML views and PDF downloads.
- Download Citation
- Rights & Permissions
top
Abstract
Figures and Tables
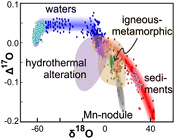
 Figure 1 Characteristic δ′18O – Δ′17O fields for different materials. Meteoric waters have uniquely high Δ′17O values and generally low δ′18O values. Marine carbonates and silica plot in a narrow band of high δ′18O and low Δ′17O values. Manganese oxides have unusually low Δ′17O values due to incorporation of dissolved O2. Hydrothermal alteration drives igneous and metamorphic rocks to lower δ′18O values. All data are standardised to VSMOW, where solid samples are normalised to Δ′17O of San Carlos olivine = -0.05 ‰. See Figure S-1 for data sources. | 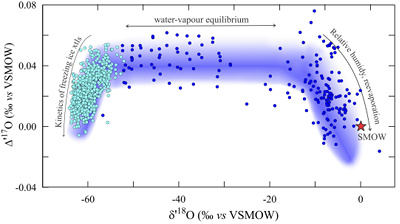 Figure 2 δ′18O – Δ′17O values of meteoric waters. Waters can be divided into three segments: extreme low values with a λ of 0.5309 (Vostok ice core - pale blue symbols); light waters with δ′18O values between -60 and -30 ‰ from high latitudes and a λ of 0.528; and heavier waters (>-20 ‰) with a best fit λ of 0.5263. | 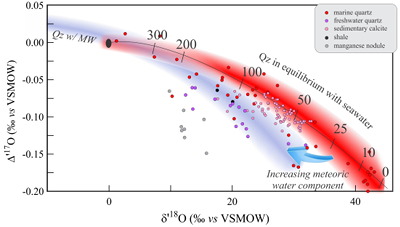 Figure 3 δ′18O – Δ′17O plot of sedimentary materials. The red silica samples equilibrated with ocean water plot along the equilibrium fractionation curved line (Sharp et al., 2016) (tick marks indicate equilibrium temperature). Samples equilibrated with meteoric water plot to the left of the marine data, consistent with a lower δ′18O and higher Δ′17O water value. Manganese oxide data plot with anomalously low Δ′17O values, suggestive of incorporation of dissolved O2 (Mandernack et al., 1995). | 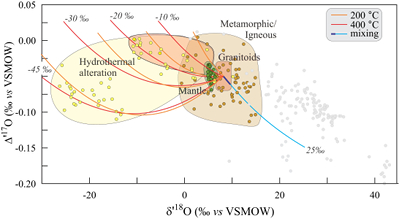 Figure 4 δ′18O – Δ′17O values of hydrothermally altered samples (pale yellow field). Calculated alteration paths are shown for a typical granite (δ′18O = 8.5 ‰, Δ′17O = -0.05) equilibrating with different compositions of meteoric water at 200 °C (orange) and 400 °C (red). The curved line defines different fluid/rock ratios emanating from the initial rock isotope value (F/R = 0). At low fluid/rock ratios, δ′18O values decrease while Δ′17O values are essentially unchanged. Only above a fluid/rock ratio of ~1 does the Δ′17O value begin to increase. Most alteration will drive typical granitoids into the limited pale red region. Assimilation of sediments will drive samples in the direction indicated by the blue curve. However, even with 10 % assimilation, the samples will only be shifted the length of the dark blue curve. |
| Figure 1 | Figure 2 | Figure 3 | Figure 4 |
top
Introduction
The early practitioners of stable isotope geochemistry recognised that the three isotopes of oxygen followed mass-dependent rules, such that fractionation in 17O/16O was approximately half that of 18O/16O for both equilibrium and kinetic processes (Craig, 1957
Craig, H. (1957) Isotopic standards for carbon and oxygen and correction factors for mass-spectrometric analysis of carbon dioxide. Geochimica et Cosmochimica Acta 12, 133-149.
). Measuring the 17O/16O ratios therefore provided no additional information to the 18O/16O values alone. Mass-independent fractionations have since been found in primitive meteorites (Clayton et al., 1973Clayton, R.N., Grossman, L., Mayeda, T.K. (1973) A component of primitive nuclear composition in carbonaceous meteorites. Science 182, 485-488.
) and many atmospheric components such as O3, CO2, H2O2 which are explained by photochemical processes (Thiemens, 2006Thiemens, M.H. (2006) History and application of mass-independent isotope effects. Annual Review of Earth and Planetary Sciences 34, 217-262.
).With improved precision, it is now recognised that there are small, but non-zero deviations from a simple best fit line in δ17O - δ18O space that are related to mass-dependent processes (Luz and Barkan, 2010
Luz, B., Barkan, E. (2010) Variations of 17O/16O and 18O/16O in meteoric waters. Geochimica et Cosmochimica Acta 74, 6276-6286.
; Pack and Herwartz, 2014Pack, A., Herwartz, D. (2014) The triple oxygen isotope composition of the Earth mantle and Δ17O variations in terrestrial rocks. Earth and Planetary Science Letters 390, 138-145.
). As more data are collected, general trends are being observed. Here we compile the bulk of published, and our own unpublished, high precision triple oxygen isotope data and categorise distinct trends in δ′18O – Δ′17O space for different processes.top
The Terrestrial Fractionation Line and Relevant Notation
A fit through δ18O - δ17O values of terrestrial materials defines the ‘Terrestrial Fractionation Line’ (TFL) with a slope of ~½. The general expression for the TFL is
Eq. 1
δ′17O = λTFL δ′18O + γTFL
where λTFL is the best fit slope and γTFL is the y-intercept of the best fit line. Equation 1 and all data in this study are presented in the linearised notation given by δ' (see Miller, 2002
Miller, M.F. (2002) Isotopic fractionation and the quantification of 17O anomalies in the oxygen three-isotope system: an appraisal and geochemical significance. Geochimica et Cosmochimica Acta 66, 1881-1889.
and Supplementary Information). There is no ‘correct’ or unique TFL, as different equilibrium or kinetic processes result in slightly different triple isotope fractionations. For example, meteoric waters fall on a trend that does not exactly overlap with the trend defined by most rocks and minerals (Luz and Barkan, 2010Luz, B., Barkan, E. (2010) Variations of 17O/16O and 18O/16O in meteoric waters. Geochimica et Cosmochimica Acta 74, 6276-6286.
; Pack and Herwartz, 2014Pack, A., Herwartz, D. (2014) The triple oxygen isotope composition of the Earth mantle and Δ17O variations in terrestrial rocks. Earth and Planetary Science Letters 390, 138-145.
; Pack et al., 2016Pack, A., Tanaka, R., Hering, M., Sengupta, S., Peters, S., Nakamura, E. (2016) The oxygen isotope composition of San Carlos olivine on the VSMOW2-SLAP2 scale. Rapid Communications in Mass Spectrometry 30, 1495-1504.
). A δ′17O - δ′18O plot of published data from natural samples is shown in Figure S-1. Virtually all data plot exactly on the same line at the resolution of the figure.To better visualise subtle deviations from the TFL reference line, the Δ′17O value has been introduced. The Δ′17O term is defined as
Eq. 2
Δ′17O = δ′17O - λRL δ′18O + γRL
λRL is the reference line slope and γRL is the y intercept. In this study, δ′17O and δ′18O values from different publications are normalised to VSMOW (rocks and minerals) and VSMOW/SLAP2 scale (waters; SLAP2 from Schoenemann et al., 2013
Schoenemann, S.W., Schauer, A.J., Steig, E.J. (2013) Measurement of SLAP2 and GISP δ17O and proposed VSMOW-SLAP normalization for δ17O and 17Oexcess. Rapid Communications in Mass Spectrometry 27, 582-590.
) and derived Δ′17O to a reference line with slope 0.528 (λRL) and zero intercept (γRL = 0). Rock samples are normalised to the VSMOW scale with the Δ′17O value of the broadly adopted San Carlos Olivine standard (SCO) having δ′18O and Δ′17O values of 5.4 ‰ and -0.05 ‰ relative to a λRL = 0.528 and γRL = 0 (Sharp et al., 2016Sharp, Z.D., Gibbons, J.A., Maltsev, O., Atudorei, V., Pack, A., Sengupta, S., Shock, E.L., Knauth, L.P. (2016) A calibration of the triple oxygen isotope fractionation in the SiO2 - H2O system and applications to natural samples. Geochimica et Cosmochimica Acta 186, 105-119.
). See Pack et al. (2016)Pack, A., Tanaka, R., Hering, M., Sengupta, S., Peters, S., Nakamura, E. (2016) The oxygen isotope composition of San Carlos olivine on the VSMOW2-SLAP2 scale. Rapid Communications in Mass Spectrometry 30, 1495-1504.
for details of normalisation.Small variations in Δ′17O become apparent in a Δ′17O vs. δ′18O plot (Fig. 1). Waters have positive Δ′17O values, while rock samples have negative Δ′17O values that tend to decrease with increasing δ′18O. Hydrothermally altered samples have the lowest δ′18O values of any rock samples.

Figure 1 Characteristic δ′18O – Δ′17O fields for different materials. Meteoric waters have uniquely high Δ′17O values and generally low δ′18O values. Marine carbonates and silica plot in a narrow band of high δ′18O and low Δ′17O values. Manganese oxides have unusually low Δ′17O values due to incorporation of dissolved O2. Hydrothermal alteration drives igneous and metamorphic rocks to lower δ′18O values. All data are standardised to VSMOW, where solid samples are normalised to Δ′17O of San Carlos olivine = -0.05 ‰. See Figure S-1 for data sources.
top
Characteristic Δ′17O - δ′18O Fields and Trends
A number of generalisations can be made from the data shown in Figure 1. A clear separation in the Δ′17O values between waters and rocks is immediately apparent. The mantle has a very tight range of Δ′17O values of -0.03 to -0.07 ‰, with most samples plotting at -0.05 ± 0.01 ‰. The 0.04 spread in Δ′17O in mantle samples is due to mantle metasomatism or alteration and in some cases analytical error. True variability within pristine mantle is likely much smaller and is centered on Δ′17O = -0.05 ‰.
The δ′18O values of more evolved high silica igneous rocks are higher than the typical mantle value of ~5.4 ‰, but the Δ′17O values remain relatively constant. This is because 1) the shift in δ′18O is small and 2) the associated high-T θ value (where θ = ln(α17O) / ln(α18O); see Supplementary Information) for minerals and melts is close to the reference slope value of 0.528, thereby moving more siliceous samples to higher δ′18O, but constant Δ′17O values.
Meteoric waters
Meteoric water data vary greatly in Δ′17O - δ′18O space (Fig. 2). Samples with δ18O values between -55 and -15 ‰ have an average Δ′17O value of 0.041 ± 0.006 and fall on a line with slope λ = 0.528. The offset in Δ′17O relative to the value of 0 ‰ for the ocean is related to the kinetic fractionation associated by transport of vapour from the saturated layer into the free, undersaturated atmosphere (Luz and Barkan, 2010
Luz, B., Barkan, E. (2010) Variations of 17O/16O and 18O/16O in meteoric waters. Geochimica et Cosmochimica Acta 74, 6276-6286.
). Samples from the Vostok ice core (pale blue points in Fig. 2) are fit with a higher λ value of 0.5309 ± 0.0001, which is attributed loosely to variable humidity and wind speed at the ocean source and supersaturation conditions during condensation in extremely cold environments (Landais et al., 2008Landais, A., Barkan, E., Luz, B. (2008) Record of δ18O and 17O-excess in ice from Vostok Antarctica during the last 150,000 years. Geophysical Research Letters 35, doi: 10.1029/2007GL032096.
) or direct condensation as ‘diamond dust’ (Miller, 2018Miller, M.F. (2018) Precipitation regime influence on oxygen triple-isotope distributions in Antarctic precipitation and ice cores. Earth and Planetary Science Letters 481, 316-327.
). For δ′18O values greater than -20 ‰, the Δ′17O values of meteoric waters show the reverse trend, decreasing with increasing δ′18O. Rain re-evaporation and mixing have been explained as the principle driving force towards the low Δ′17O values (Landais et al., 2010Landais, A., Risi, C., Bony, S., Vimeux, F., Descroix, L., Falourd, S., Bouygues, A. (2010) Combined measurements of 17Oexcess and d-excess in African monsoon precipitation: Implications for evaluating convective parameterizations. Earth and Planetary Science Letters 298, 104-112.
; Risi et al., 2013Risi, C., Landais, A., Winkler, R., Vimeux, F. (2013) Can we determine what controls the spatio-temporal distribution of d-excess and 17O-excess in precipitation using the LMDZ general circulation model. Climate of the Past 9, 2173-2193.
; Li et al., 2015Li, S., Levin, N.E., Chesson, L.A. (2015) Continental scale variation in 17O-excess of meteoric waters in the United States. Geochimica et Cosmochimica Acta 164, 110-126.
).The best fit line to meteoric water samples with a δ′18O value greater than -20 ‰ is
Eq. 3
δ′17O = 0.52654 (±0.00036) δ′18O + 0.014 (±0.003).
Most waters outside of extreme polar regions have δ18O values that are greater than ‑20 ‰, so that Equation 3 is a rough fit to the Global Meteoric Water Line for waters from non-polar sample regions.

Figure 2 δ′18O – Δ′17O values of meteoric waters. Waters can be divided into three segments: extreme low values with a λ of 0.5309 (Vostok ice core - pale light blue symbols); light waters with δ′18O values between -60 and -30 ‰ from high latitudes and a λ of 0.528; and heavier waters (>-20 ‰) with a best fit λ of 0.5263.
Low temperature sediments
A compilation of data from low temperature sediments shows decreasing Δ′17O values with increasing δ′18O (Fig. 3). This trend is explained by the temperature effect on the equilibrium fractionation between minerals and seawater. The triple isotope equilibrium θ value decreases with decreasing temperature, (Cao and Liu, 2011
Cao, X., Liu, Y. (2011) Equilibrium mass-dependent fractionation relationships for triple oxygen isotopes. Geochimica et Cosmochimica Acta 75, 7435-7445.
; Sharp et al., 2016Sharp, Z.D., Gibbons, J.A., Maltsev, O., Atudorei, V., Pack, A., Sengupta, S., Shock, E.L., Knauth, L.P. (2016) A calibration of the triple oxygen isotope fractionation in the SiO2 - H2O system and applications to natural samples. Geochimica et Cosmochimica Acta 186, 105-119.
). The curved line in Figure 3 is the equation governing the Δ′17O - δ′18O values of silica in equilibrium with ocean water as a function of temperature (Sharp et al., 2016Sharp, Z.D., Gibbons, J.A., Maltsev, O., Atudorei, V., Pack, A., Sengupta, S., Shock, E.L., Knauth, L.P. (2016) A calibration of the triple oxygen isotope fractionation in the SiO2 - H2O system and applications to natural samples. Geochimica et Cosmochimica Acta 186, 105-119.
). Most marine silica samples fall on or near this curved line. Samples that have equilibrated with meteoric waters consequently plot towards lower δ′18O and, in general, higher Δ′17O values, consistent with a lighter meteoric water source. Our unpublished manganese oxide data from a deep sea nodule off Hawaii have very low Δ′17O values in relation to their δ′18O values, explained by incorporation of dissolved air O2 into the MnO2 structure (Δ′17Oair = -0.41 to -0.45). The low Δ′17O values support the conclusion that dissolved O2 is directly incorporated in manganates during biogenic and abiogenic laboratory synthesis experiments (Mandernack et al., 1995Mandernack, K.W., Fogel, M.L., Tebo, B.M., Usui, A. (1995) Oxygen isotope analyses of chemically and microbially produced manganese oxides and manganates. Geochimica et Cosmochimica Acta 59, 4409-4425.
).
Figure 3 δ′18O – Δ′17O plot of sedimentary materials. The red silica samples equilibrated with ocean water plot along the equilibrium fractionation curved line (Sharp et al., 2016
Sharp, Z.D., Gibbons, J.A., Maltsev, O., Atudorei, V., Pack, A., Sengupta, S., Shock, E.L., Knauth, L.P. (2016) A calibration of the triple oxygen isotope fractionation in the SiO2 - H2O system and applications to natural samples. Geochimica et Cosmochimica Acta 186, 105-119.
) (tick marks indicate equilibrium temperature). Samples equilibrated with meteoric water plot to the left of the marine data, consistent with a lower δ′18O and higher Δ′17O water value. Manganese oxide data plot with anomalously low Δ′17O values, suggestive of incorporation of dissolved O2 (Mandernack et al., 1995Mandernack, K.W., Fogel, M.L., Tebo, B.M., Usui, A. (1995) Oxygen isotope analyses of chemically and microbially produced manganese oxides and manganates. Geochimica et Cosmochimica Acta 59, 4409-4425.
).Hydrothermal mixing
A number of variables control the isotopic effect during hydrothermal alteration, including temperature, initial rock protolith and fluid compositions, and fluid/rock (F/R) ratio. Nevertheless, the δ′18O - Δ′17O field of most hydrothermally altered rock is surprisingly limited.
Hydrothermal alteration is modelled using a simple mass balance mixing equation. A fraction of water is allowed to equilibrate with a rock at a given temperature. The bulk composition is given by
Eq. 4
δ18Obulk = Xwater (δ18Owater initial) + (1-Xwater) (δ18Orock initial) = Xwater (δ18Owater final) + (1-Xwater) (δ18Orock final)
The final δ18O value of the rock is determined by the additional fractionation equation
Eq. 5
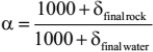
where α varies with temperature.
Mixing calculations are made using the standard delta values. Once the final isotopic compositions are determined, data are linearised for plotting on δ′18O – Δ′17O plots. In linearised plots, mixing trends plot as curved lines.
Figure 4 shows mixing trends between a typical unaltered granitoid (δ′18O = 8.5 ‰; Δ′17O = -0.05 ‰) and meteoric waters of varying composition. Fractionation factors for this example are for quartz. As fluid/rock ratios increase, the delta values of the altered rock initially move to lower δ′18O and ultimately higher Δ′17O values. An extreme example from the ‘snowball Earth’ altered samples from Karelia (Herwartz et al., 2015
Herwartz, D., Pack, A., Krylov, D., Xiao, Y., Muehlenbachs, K., Sengupta, S., Di Rocco, T. (2015) Revealing the climate of snowball Earth from Δ17O systematics of hydrothermal rocks. Proceedings of the National Academy of Sciences 112, 5337-5341.
) results in δ′18O values as low as -26 ‰, vastly expanding the hydrothermal alteration field (yellow field in Fig. 4). In more common cases where the δ18O value of meteoric water is -20 ‰ or higher, the hydrothermal alteration box is far more limited (red field in Fig. 4).Assimilation of a high δ18O sediment by a granitoid will shift the δ′18O values higher, and the Δ′17O values lower (pale blue curve in Fig. 4). In practice, the effect will be small. Even 10 % incorporation of sediment will only move the altered rock as far as the dark blue curve.

Figure 4 δ′18O – Δ′17O values of hydrothermally altered samples (pale yellow field). Calculated alteration paths are shown for a typical granite (δ′18O = 8.5 ‰, Δ′17O = -0.05) equilibrating with different compositions of meteoric water at 200 °C (orange) and 400 °C (red). The curved line defines different fluid/rock ratios emanating from the initial rock isotope value (F/R = 0). At low fluid/rock ratios, δ′18O values decrease while Δ′17O values are essentially unchanged. Only above a fluid/rock ratio of ~1 does the Δ′17O value begin to increase. Most alteration will drive typical granitoids into the limited pale red region. Assimilation of sediments will drive samples in the direction indicated by the blue curve. However, even with 10 % assimilation, the samples will only be shifted the length of the dark blue curve.
top
General Fields and Choice of λRL
Figure 1 shows the characteristic δ′18O – Δ′17O regions for different materials. While there is some overlap, different rock types generally plot in distinct regions following well established fractionation rules. The best fit λ value depends on which samples are considered (Fig. S-2). All data have a best fit λ = 0.5269. Waters define a λ value of 0.5281, but if the light Vostok ice core samples are excluded, the λ becomes 0.5275. Rocks have a still smaller λ value, controlled in large part by the high δ′18O – low Δ′17O sedimentary samples.
The isotope community in this nascent field has not reached consensus on a standard reference line ‒ the λRL value ‒ for calculating Δ¢17O values. We suggest reporting both δ17O and δ18O on VSMOW scale. For materials with δ values departing far from zero, one may apply VSMOW/SLAP2 scaling. This ensures that data from the rock and water community remain comparable.
The meteorological community uses a λRL value of 0.528, as this initially had been suggested to be representative of the Global Meteoric Water Line slope (Luz and Barkan, 2010
Luz, B., Barkan, E. (2010) Variations of 17O/16O and 18O/16O in meteoric waters. Geochimica et Cosmochimica Acta 74, 6276-6286.
). With such a definition, most rocks have negative Δ¢17O values, whereas meteoric waters generally have positive values. Some studies adopt a λRL = 0.5305, the theoretical θ value at infinite temperature. All natural, equilibrium processes should have θ values less than this value, so that trends for all data should have a negative slope in Δ′17O - δ′18O space. In practice, some materials following complex kinetic processes have λ values higher than 0.5305, notably the Vostok ice core data (λ = 0.5309 ± 0.0002) and precipitation from Niger (Landais et al., 2010Landais, A., Risi, C., Bony, S., Vimeux, F., Descroix, L., Falourd, S., Bouygues, A. (2010) Combined measurements of 17Oexcess and d-excess in African monsoon precipitation: Implications for evaluating convective parameterizations. Earth and Planetary Science Letters 298, 104-112.
) (λ = 0.543 ± 0.004). Most processes undergo fractionation with a θ value (significantly less than 0.5305), resulting in a strong negative trend in Δ′17O as a function of δ′18O in this reference frame (Fig. S-3). The adoption of λRL = 0.5305 is consistent with other multiple isotope systems, where the theoretical high-T limit is used as a reference. A λRL = 0.528 would make the ‘rock’ data compatible with the longstanding data on meteoric waters and would not artificially skew Δ′17O to more negative values with increasing δ′18O values. This is critical as researchers combine ‘water’ and ‘rock’ data. The authors of this contribution do not agree on a suggested convention.top
Conclusions
The δ′17O - δ′18O values for all terrestrial materials not affected by photochemical effects plot on a straight line with a slope of ~½. In detail, small, purely mass-dependent variations can be resolved and displayed in Δ′17O - δ′18O space (Fig. 1). The vast majority of meteoric waters have positive Δ′17O values, while rocks have negative Δ′17O values. Low- and high-T rocks fall on distinct trends and fields that can be explained by well understood processes. It is unlikely that many data will fall to the right hand side of the seawater - silica equilibrium curve nor are many rocks expected to fall above the meteoric water line. These may be termed "forbidden" regions. Similarly, samples with Δ′17O values less than -0.10 are probably unique to low temperature sediments (or products of low temperature sediments). The distinction between the different regions outlined here will undoubtedly show more overlap as additional data are collected. Overall, however, the combined δ′18O - Δ′17O values of rocks and waters are characteristic of their formation conditions.
top
Author Contributions and Acknowledgements
All authors contributed ideas and helped in writing the final manuscript. JW provided unpublished calcite isotope data. We acknowledge E. Cano for unpublished data for mantle samples and two anonymous reviewers.
Editor: Eric H. Oelkers
top
References
Cao, X., Liu, Y. (2011) Equilibrium mass-dependent fractionation relationships for triple oxygen isotopes. Geochimica et Cosmochimica Acta 75, 7435-7445.
 Show in context
Show in contextThe triple isotope equilibrium θ value decreases with decreasing temperature, (Cao and Liu, 2011; Sharp et al., 2016).
View in article
Clayton, R.N., Grossman, L., Mayeda, T.K. (1973) A component of primitive nuclear composition in carbonaceous meteorites. Science 182, 485-488.
 Show in context
Show in contextMass-independent fractionations have since been found in primitive meteorites (Clayton et al., 1973) and many atmospheric components such as O3, CO2, H2O2 which are explained by photochemical processes (Thiemens, 2006).
View in article
Craig, H. (1957) Isotopic standards for carbon and oxygen and correction factors for mass-spectrometric analysis of carbon dioxide. Geochimica et Cosmochimica Acta 12, 133-149.
 Show in context
Show in context The early practitioners of stable isotope geochemistry recognised that the three isotopes of oxygen followed mass-dependent rules, such that fractionation in 17O/16O was approximately half that of 18O/16O for both equilibrium and kinetic processes (Craig, 1957).
View in article
Herwartz, D., Pack, A., Krylov, D., Xiao, Y., Muehlenbachs, K., Sengupta, S., Di Rocco, T. (2015) Revealing the climate of snowball Earth from Δ17O systematics of hydrothermal rocks. Proceedings of the National Academy of Sciences 112, 5337-5341.
 Show in context
Show in context An extreme example from the ‘snowball Earth’ altered samples from Karelia (Herwartz et al., 2015) results in δ′18O values as low as -26 ‰, vastly expanding the hydrothermal alteration field (yellow field in Fig. 4).
View in article
Landais, A., Barkan, E., Luz, B. (2008) Record of δ18O and 17O-excess in ice from Vostok Antarctica during the last 150,000 years. Geophysical Research Letters 35, doi: 10.1029/2007GL032096.
 Show in context
Show in contextSamples from the Vostok ice core (pale blue points in Fig. 2) are fit with a higher λ value of 0.5309 ± 0.0001, which is attributed loosely to variable humidity and wind speed at the ocean source and supersaturation conditions during condensation in extremely cold environments (Landais et al., 2008) or direct condensation as ‘diamond dust’ (Miller, 2018).
View in article
Landais, A., Risi, C., Bony, S., Vimeux, F., Descroix, L., Falourd, S., Bouygues, A. (2010) Combined measurements of 17Oexcess and d-excess in African monsoon precipitation: Implications for evaluating convective parameterizations. Earth and Planetary Science Letters 298, 104-112.
 Show in context
Show in context Rain re-evaporation and mixing have been explained as the principle driving force towards the low Δ′17O values (Landais et al., 2010; Risi et al., 2013; Li et al., 2015).
View in article
In practice, some materials following complex kinetic processes have λ values higher than 0.5305, notably the Vostok ice core data (λ = 0.5309 ± 0.0002) and precipitation from Niger (Landais et al., 2010) (λ = 0.543 ± 0.004).
View in article
Li, S., Levin, N.E., Chesson, L.A. (2015) Continental scale variation in 17O-excess of meteoric waters in the United States. Geochimica et Cosmochimica Acta 164, 110-126.
 Show in context
Show in contextRain re-evaporation and mixing have been explained as the principle driving force towards the low Δ′17O values (Landais et al., 2010; Risi et al., 2013; Li et al., 2015).
View in article
Luz, B., Barkan, E. (2010) Variations of 17O/16O and 18O/16O in meteoric waters. Geochimica et Cosmochimica Acta 74, 6276-6286.
 Show in context
Show in context With improved precision, it is now recognised that there are small, but non-zero deviations from a simple best fit line in δ17O - δ18O space that are related to mass-dependent processes (Luz and Barkan, 2010; Pack and Herwartz, 2014).
View in article
For example, meteoric waters fall on a trend that does not exactly overlap with the trend defined by most rocks and minerals (Luz and Barkan, 2010; Pack and Herwartz, 2014; Pack et al., 2016).
View in article
The offset in Δ′17O relative to the value of 0 ‰ for the ocean is related to the kinetic fractionation associated by transport of vapour from the saturated layer into the free, undersaturated atmosphere (Luz and Barkan, 2010).
View in article
The meteorological community uses a λRL value of 0.528, as this initially had been suggested to be representative of the Global Meteoric Water Line slope (Luz and Barkan, 2010).
View in article
Mandernack, K.W., Fogel, M.L., Tebo, B.M., Usui, A. (1995) Oxygen isotope analyses of chemically and microbially produced manganese oxides and manganates. Geochimica et Cosmochimica Acta 59, 4409-4425.
 Show in context
Show in contextThe low Δ′17O values support the conclusion that dissolved O2 is directly incorporated in manganates during biogenic and abiogenic laboratory synthesis experiments (Mandernack et al., 1995).
View in article
Figure 3 [...] Manganese oxide data plot with anomalously low Δ′17O values, suggestive of incorporation of dissolved O2 (Mandernack et al., 1995).
View in article
Miller, M.F. (2002) Isotopic fractionation and the quantification of 17O anomalies in the oxygen three-isotope system: an appraisal and geochemical significance. Geochimica et Cosmochimica Acta 66, 1881-1889.
 Show in context
Show in contextEquation 1 and all data in this study are presented in the linearised notation given by δ' (see Miller, 2002 and Supplementary Information).
View in article
Miller, M.F. (2018) Precipitation regime influence on oxygen triple-isotope distributions in Antarctic precipitation and ice cores. Earth and Planetary Science Letters 481, 316-327.
 Show in context
Show in context Samples from the Vostok ice core (pale blue points in Fig. 2) are fit with a higher λ value of 0.5309 ± 0.0001, which is attributed loosely to variable humidity and wind speed at the ocean source and supersaturation conditions during condensation in extremely cold environments (Landais et al., 2008) or direct condensation as ‘diamond dust’ (Miller, 2018).
View in article
Pack, A., Herwartz, D. (2014) The triple oxygen isotope composition of the Earth mantle and Δ17O variations in terrestrial rocks. Earth and Planetary Science Letters 390, 138-145.
 Show in context
Show in contextWith improved precision, it is now recognised that there are small, but non-zero deviations from a simple best fit line in δ17O - δ18O space that are related to mass-dependent processes (Luz and Barkan, 2010; Pack and Herwartz, 2014).
View in article
For example, meteoric waters fall on a trend that does not exactly overlap with the trend defined by most rocks and minerals (Luz and Barkan, 2010; Pack and Herwartz, 2014; Pack et al., 2016).
View in article
Pack, A., Tanaka, R., Hering, M., Sengupta, S., Peters, S., Nakamura, E. (2016) The oxygen isotope composition of San Carlos olivine on the VSMOW2-SLAP2 scale. Rapid Communications in Mass Spectrometry 30, 1495-1504.
 Show in context
Show in context For example, meteoric waters fall on a trend that does not exactly overlap with the trend defined by most rocks and minerals (Luz and Barkan, 2010; Pack and Herwartz, 2014; Pack et al., 2016).
View in article
See Pack et al. (2016) for details of normalisation.
View in article
Risi, C., Landais, A., Winkler, R., Vimeux, F. (2013) Can we determine what controls the spatio-temporal distribution of d-excess and 17O-excess in precipitation using the LMDZ general circulation model. Climate of the Past 9, 2173-2193.
 Show in context
Show in contextRain re-evaporation and mixing have been explained as the principle driving force towards the low Δ′17O values (Landais et al., 2010; Risi et al., 2013; Li et al., 2015).
View in article
Schoenemann, S.W., Schauer, A.J., Steig, E.J. (2013) Measurement of SLAP2 and GISP δ17O and proposed VSMOW-SLAP normalization for δ17O and 17Oexcess. Rapid Communications in Mass Spectrometry 27, 582-590.
 Show in context
Show in context In this study, δ′17O and δ′18O values from different publications are normalised to VSMOW (rocks and minerals) and VSMOW/SLAP2 scale (waters; SLAP2 from Schoenemann et al., 2013) and derived Δ′17O to a reference line with slope 0.528 (λRL) and zero intercept (γRL = 0).
View in article
Sharp, Z.D., Gibbons, J.A., Maltsev, O., Atudorei, V., Pack, A., Sengupta, S., Shock, E.L., Knauth, L.P. (2016) A calibration of the triple oxygen isotope fractionation in the SiO2 - H2O system and applications to natural samples. Geochimica et Cosmochimica Acta 186, 105-119.
 Show in context
Show in contextRock samples are normalised to the VSMOW scale with the Δ′17O value of the broadly adopted San Carlos Olivine standard (SCO) having δ′18O and Δ′17O values of 5.4 ‰ and -0.05 ‰ relative to a λRL = 0.528 and γRL = 0 (Sharp et al., 2016).
View in article
The triple isotope equilibrium θ value decreases with decreasing temperature, (Cao and Liu, 2011; Sharp et al., 2016).
View in article
The curved line in Figure 3 is the equation governing the Δ′17O - δ′18O values of silica in equilibrium with ocean water as a function of temperature (Sharp et al., 2016).
View in article
Figure 3 [...] The red silica samples equilibrated with ocean water plot along the equilibrium fractionation curved line (Sharp et al., 2016) (tick marks indicate equilibrium temperature).
View in article
Thiemens, M.H. (2006) History and application of mass-independent isotope effects. Annual Review of Earth and Planetary Sciences 34, 217-262.
 Show in context
Show in contextMass-independent fractionations have since been found in primitive meteorites (Clayton et al., 1973) and many atmospheric components such as O3, CO2, H2O2 which are explained by photochemical processes (Thiemens, 2006).
View in article
top
Supplementary Information
The Supplementary Information includes:
- Fractionation Equations
- The Terrestrial Fractionation Line and the Definition of Δ′17O
- Figures S-1 to S-3
- Supplementary Information References
Download the Supplementary Information (PDF).
Figures and Tables
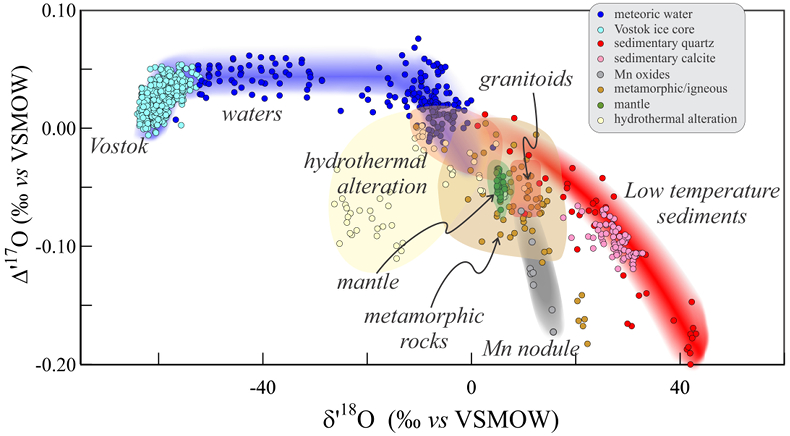
Figure 1 Characteristic δ′18O – Δ′17O fields for different materials. Meteoric waters have uniquely high Δ′17O values and generally low δ′18O values. Marine carbonates and silica plot in a narrow band of high δ′18O and low Δ′17O values. Manganese oxides have unusually low Δ′17O values due to incorporation of dissolved O2. Hydrothermal alteration drives igneous and metamorphic rocks to lower δ′18O values. All data are standardised to VSMOW, where solid samples are normalised to Δ′17O of San Carlos olivine = -0.05 ‰. See Figure S-1 for data sources.
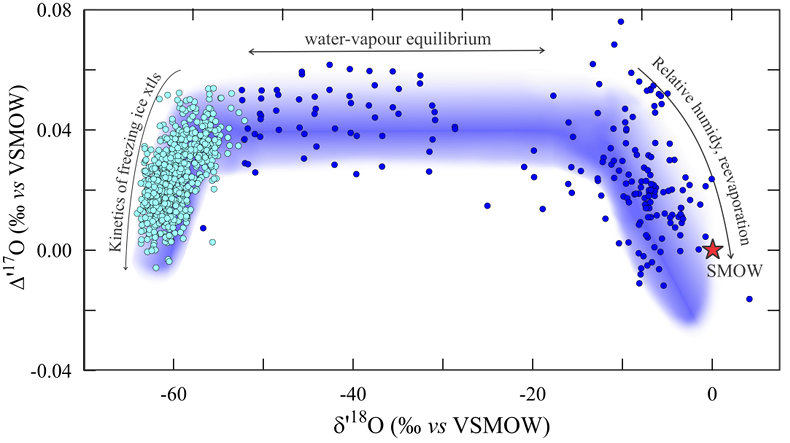
Figure 2 δ′18O – Δ′17O values of meteoric waters. Waters can be divided into three segments: extreme low values with a λ of 0.5309 (Vostok ice core - pale blue symbols); light waters with δ′18O values between -60 and -30 ‰ from high latitudes and a λ of 0.528; and heavier waters (>-20 ‰) with a best fit λ of 0.5263.
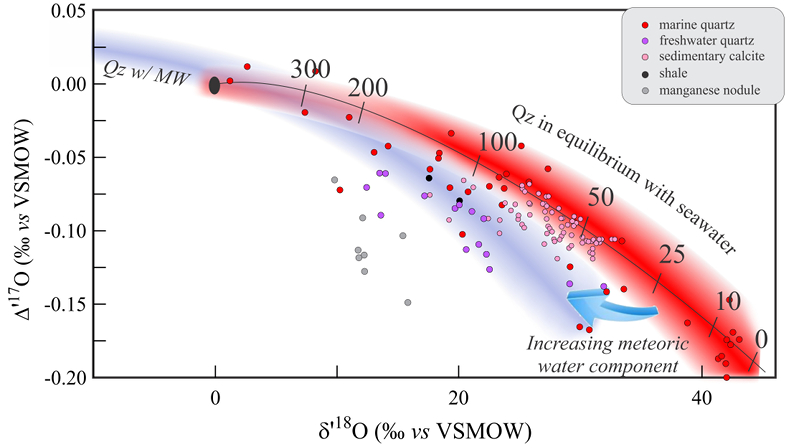
Figure 3 δ′18O – Δ′17O plot of sedimentary materials. The red silica samples equilibrated with ocean water plot along the equilibrium fractionation curved line (Sharp et al., 2016
Sharp, Z.D., Gibbons, J.A., Maltsev, O., Atudorei, V., Pack, A., Sengupta, S., Shock, E.L., Knauth, L.P. (2016) A calibration of the triple oxygen isotope fractionation in the SiO2 - H2O system and applications to natural samples. Geochimica et Cosmochimica Acta 186, 105-119.
) (tick marks indicate equilibrium temperature). Samples equilibrated with meteoric water plot to the left of the marine data, consistent with a lower δ′18O and higher Δ′17O water value. Manganese oxide data plot with anomalously low Δ′17O values, suggestive of incorporation of dissolved O2 (Mandernack et al., 1995Mandernack, K.W., Fogel, M.L., Tebo, B.M., Usui, A. (1995) Oxygen isotope analyses of chemically and microbially produced manganese oxides and manganates. Geochimica et Cosmochimica Acta 59, 4409-4425.
).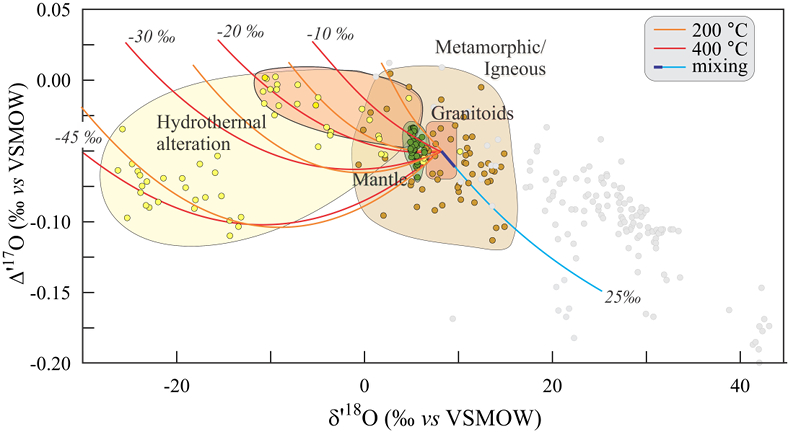
Figure 4 δ′18O – Δ′17O values of hydrothermally altered samples (pale yellow field). Calculated alteration paths are shown for a typical granite (δ′18O = 8.5 ‰, Δ′17O = -0.05) equilibrating with different compositions of meteoric water at 200 °C (orange) and 400 °C (red). The curved line defines different fluid/rock ratios emanating from the initial rock isotope value (F/R = 0). At low fluid/rock ratios, δ′18O values decrease while Δ′17O values are essentially unchanged. Only above a fluid/rock ratio of ~1 does the Δ′17O value begin to increase. Most alteration will drive typical granitoids into the limited pale red region. Assimilation of sediments will drive samples in the direction indicated by the blue curve. However, even with 10 % assimilation, the samples will only be shifted the length of the dark blue curve.